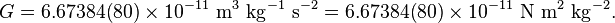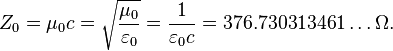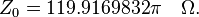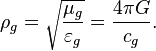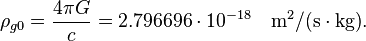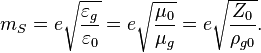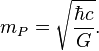Vacuum constants

Vacuum constants are physical constants associated with the fields existing in the free space under high vacuum. The values of these constants can be determined from the analysis of the interaction of fields with matter. Vacuum constants come in a variety of physical equations as necessary coefficients. Because of this, great importance is the refinement of these constants in special experiments.

Basic constants

Speed of light: ^[1] 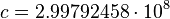$c = 2.99792458\cdot 10^8 \$ m/s, as exact value. It has become a defined constant in the SI system of units.

Vacuum permittivity: ^[2] 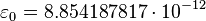$\varepsilon_0 = 8.854187817\cdot 10^{-12} \$ F/m.

Speed of gravity 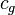$c_g$ . It is supposed that 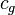$c_g$ equals to the speed of light.

Gravitational constant: $G = 6.67384(80) \times 10^{-11} \ \rm{m}^3 \ \rm{kg}^{-1} \ \rm{s}^{-2} = 6.67384(80) \times 10^{-11} \ {\rm N} \ {\rm m^{2}} \ {\rm kg^{-2}}.$

Derivative constants

Vacuum permeability: 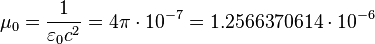$\mu_0 = \frac{1}{\varepsilon_0 c^2}=4 \pi \cdot 10^{-7} = 1.2566370614 \cdot 10^{-6} \$ H/m in the SI system of units.

Electromagnetic impedance of free space:

Z_0 = \mu_0 c = \sqrt{\frac{\mu_0}{\varepsilon_0}}= \frac{1}{\varepsilon_0 c} = 376.730313461 \ldots \Omega.

Since $\mu_0$ and $c$ have exact values the same is for impedance of free space:

Z_0 = 119.9169832 \pi \quad \Omega.

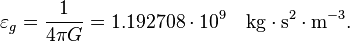
Gravitoelectric gravitational constant: $~\varepsilon_g = \frac{1}{4\pi G } = 1.192708\cdot 10^9 \quad \mathrm {kg \cdot s^2 \cdot m^{-3}}.$

Gravitomagnetic gravitational constant: 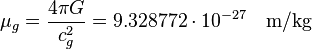$~\mu_g = \frac{4\pi G }{ c^2_g} = 9.328772\cdot 10^{-27}\quad \mathrm {m / kg}$ , if $c_g =c$ .

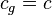Gravitational characteristic impedance of free space:

~\rho_{g} =\sqrt{\frac{\mu_g}{\varepsilon_g}} = \frac{4\pi G }{c_g}.

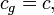If $~ c_{g}=c,$ then gravitational characteristic impedance of free space equals to: ^[3] ^[4]

~ \rho_{g0} = \frac{4\pi G }{c} =2.796696\cdot 10^{-18} \quad \mathrm {m^2/(s\cdot kg)}.

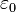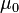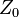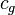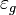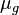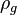Constants $c$ , $\varepsilon_0$ , $\mu_0$ and $Z_0$ belong to selfconsistent electromagnetic constants, and constants $c_g$ , $\varepsilon_g$ , $\mu_g$ and $\rho_{g}$ belong to selfconsistent gravitational constants.

Vacuum constants are used for creation of natural units such as Stoney units and Planck units. For example, Stoney mass is connected with elementary charge $e$ :

m_S = e \sqrt{\frac{\varepsilon_g}{\varepsilon_0}} = e \sqrt{\frac{\mu_0}{\mu_g}} = e \sqrt{\frac{Z_0}{\rho_{g0}}}.

The Planck mass is connected with Dirac constant 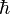$\hbar$ :

m_P = \sqrt{\frac{ \hbar c}{G}} .

The Stoney length and the Stoney energy, collectively called the Stoney scale, are not far from the Planck length and the Planck energy, the Planck scale.

References

↑ CODATA value: Speed of Light in Vacuum. The NIST reference on Constants, Units, and Uncertainty. NIST.
↑ Latest (2010) values of the constants
↑ J. D. Kraus, IEEE Antennas and Propagation. Magazine 33, 21 (1991).
↑ Raymond Y. Chiao. "New directions for gravitational wave physics via “Millikan oil drops”, arXiv:gr-qc/0610146v16 (2007).PDF

External links

Vacuum constants in Russian

This article is issued from Wikiversity - version of the Wednesday, October 29, 2014. The text is available under the Creative Commons Attribution/Share Alike but additional terms may apply for the media files.