Trigonometry/Spherical
< Trigonometry
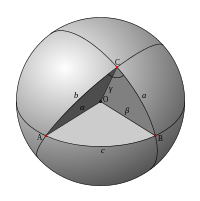
Spherical trigonometry relates to spherical triangles, and trihedral angles. A spherical triangle is a triangle projected on a sphere. Spherical trigonometry has applications in navigation, and astronomy.
Definitions and explanations
A lune is a shape on a sphere from one angle merged to an angle on the opposite side of the sphere. It is formed by two circles on a sphere. The area between two lines of longitude on one side of a globe are an example of a lune. A digon or a bi-angle are other terms for a lune.
A lune is composed of two spherical triangles, when split in half equidistant from each angle. These two congruent spherical triangles are called colunar triangles.
Unlike plane trigonometry, in spherical trigonometry it is possible for a spherical triangle to have up to three obtuse or right angles.
A birectangular triangle has two right angles.
A trirectangular triangle has three right angles.
Polar triangle are triangles with angles at the center of the sphere, and they are in relation to spherical triangles.
References
- Moritz, Robert (1913), A Text-book on Spherical Trigonometry (First ed.), John Wiley and Sons
- Wikipedia: Spherical Trigonometry