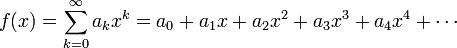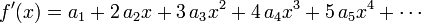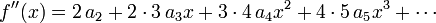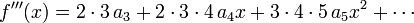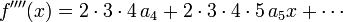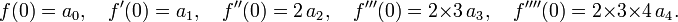Taylor's series

A well-behaved function can be expanded into a power series. This means that for all non-negative integers 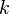$k$ there are real numbers 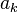$a_k$ such that

f(x)=\sum_{k=0}^\infty a_kx^k=a_0+a_1x+a_2x^2+a_3x^3+a_4x^4+\cdots

Let us calculate the first four derivatives using 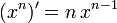$(x^n)'=n\,x^{n-1}$ :

f'(x)=a_1+2\,a_2x+3\,a_3x^2+4\,a_4x^3+5\,a_5x^4+\cdots

f''(x)=2\,a_2+2\cdot3\,a_3x+3\cdot4\,a_4x^2+4\cdot5\,a_5x^3+\cdots

f'''(x)=2\cdot3\,a_3+2\cdot3\cdot4\,a_4x+3\cdot4\cdot5\,a_5x^2+\cdots

f''''(x)=2\cdot3\cdot4\,a_4+2\cdot3\cdot4\cdot5\,a_5x+\cdots

Setting 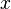$x$ equal to zero, we obtain

f(0)=a_0,\quad f'(0)=a_1,\quad f''(0)=2\,a_2,\quad f'''(0)=2\times3\,a_3,\quad f''''(0)=2\times3\times4\,a_4.

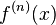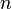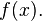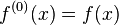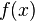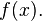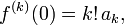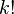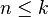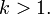Let us write $f^{(n)}(x)$ for the $n$ -th derivative of $f(x).$ We also write $f^{(0)}(x)=f(x)$ — think of $f(x)$ as the "zeroth derivative" of $f(x).$ We thus arrive at the general result $f^{(k)}(0)=k!\,a_k,$ where the factorial $k!$ is defined as equal to 1 for $k=0$ and $k=1$ and as the product of all natural numbers $n\leq k$ for $k>1.$ Expressing the coefficients 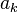$a_k$ in terms of the derivatives of 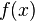$f(x)$ at $x=0,$ 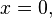we obtain

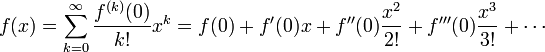f(x)=\sum_{k=0}^\infty {f^{(k)}(0)\over k!}x^k=f(0)+f'(0)x+f''(0){x^2\over2!}+f'''(0){x^3\over3!}+\cdots

This is the Taylor series for 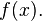$f(x).$

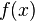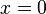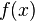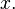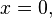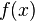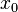A remarkable result: if you know the value of a well-behaved function $f(x)$ and the values of all of its derivatives at the single point $x=0$ then you know $f(x)$ at all points $x.$ Besides, there is nothing special about $x=0,$ so $f(x)$ is also determined by its value and the values of its derivatives at any other point $x_0$ :

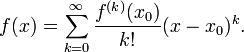f(x)=\sum_{k=0}^\infty {f^{(k)}(x_0)\over k!}(x-x_0)^k.

This article is issued from Wikiversity - version of the Sunday, July 29, 2012. The text is available under the Creative Commons Attribution/Share Alike but additional terms may apply for the media files.