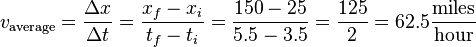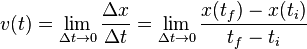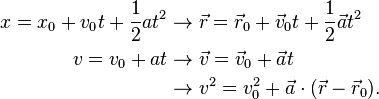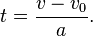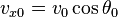Physics equations/Kinematics
< Physics equations"D" or delta as difference
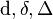 , and
, and  all mean difference :
all mean difference :
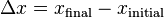 ,
,
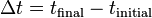 .
Other subscripts are also used (e.g.,
.
Other subscripts are also used (e.g., 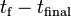 , or
, or 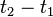 , or
, or 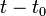 .)
.)
One dimensional velocity and acceleration
Velocity is the rate at which position changes. Acceleration is the rate at which velocity changes. If the time interval is not infinitesimally small, we refer to these as "average" rates. The average velocity or acceleration is often denoted by a bar above. Alternatives to 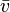 to are the brakcet
to are the brakcet 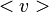 and the subscript
and the subscript 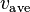 .
.
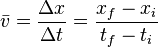 ,
, 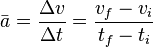 .
.
Instantaneous velocity and acceleration are derivatives: 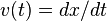 ,
, 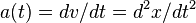
A driver gets on mile 25 of a freeway at 3:00 pm and exits at mile 150 at 5:30 pm. If the road is straight, what is the velocity and is it average or instantaneous?
It is an average velocity because the time interval is not infinitesimally small. (In physics we like to be precise and call it velocity and not speed because if the person was going in the opposite direction, the result would have been negative. Velocity has direction as a property, speed does not. [1]
Express instantaneous velocity and acceleration in the language of calculus and the derivative. By definition, velocity involves two different positions at two different times. However, we may take the limit that these differences are very small and define the instantaneous velocity. A connection to differential calculus is seen by rewriting
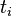 and
and 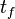 as t and t+
as t and t+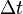 , so that
, so that 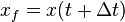 :
: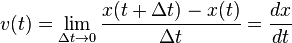
Find velocity and acceleration if 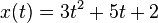
A particle's motion[2] is described by the equation
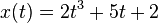 . Find a) the particle's velocity function, b) its instantaneous velocity at t = 2 s. Also find c) the particle's acceleration function and d) its instantaneous acceleration at t = 2 s.
. Find a) the particle's velocity function, b) its instantaneous velocity at t = 2 s. Also find c) the particle's acceleration function and d) its instantaneous acceleration at t = 2 s.
First we note that the coefficients need units (these units are often omitted when calculating informally):
![x(t) = 2\left [\frac{\mathrm{m}}{\mathrm{s}^3}\right ]\ t^3 +
5\left [\frac{\mathrm{m}}{\mathrm{s}^2}\right ]\ t +
2\ \mathrm{m}\](../I/m/4a61c5ce3a47636ce9c020fb65d17662.png)
a)
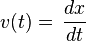
![=
\,\frac{d}{dt} \left (
2\left [\frac{\mathrm{m}}{\mathrm{s}^3}\right ]\ t^3 +
5\left [\frac{\mathrm{m}}{\mathrm{s}}\right ]\ t +
2\ \mathrm{m}\ \right )](../I/m/2ac3f25b3366e6a2553d5a27b114d42d.png)
![=
\underline{\overline{ \left |
6 \left [\frac{\mathrm{m}}{\mathrm{s}^3}\right ]\ t^2 +
5\left [\frac{\mathrm{m}}{\mathrm{s}}\right ]
\right |}}](../I/m/4182c649d73a6689cfb0f838452a2be6.png)
b)
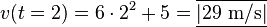
c)
![a(t) = \,\frac{d}{dt} \left (6 \left [\frac{\mathrm{m}}{\mathrm{s}^3}\right ]\ t^2 +
5\left [\frac{\mathrm{m}}{\mathrm{s}}\right ] \right ) =
\underline{\overline{ \left |
12\left [\frac{\mathrm{m}}{\mathrm{s^3}}\right ]t
\right |}}](../I/m/67f1fa0af82af3e79795ae30685f5744.png)
d)
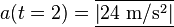
Average acceleration when reversing direction A person is jogging east at 3m/s when he suddenly reverses direction and is jogging west at 3m/s, taking one second to accomplish this reversal. What is the average acceleration? - Answer: +6m/s if west is the positive direction, and -6m/s if east is the positive direction.
Uniformly accelerated motion in one and two dimensions
The student should first master this concept in the simple notation before attempting to generalization that follows the  symbol. We shall employ this symbol to highlight how physics is often expressed using sequence of simple-to-powerful notation (click the link for further discussion).
symbol. We shall employ this symbol to highlight how physics is often expressed using sequence of simple-to-powerful notation (click the link for further discussion).
Explain the meaning of (x0,x0,a). * 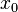 is the initial value of position (
is the initial value of position (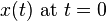
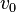 is the initial value of velocity(
is the initial value of velocity(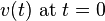
 is the acceleration (assumed constant).
is the acceleration (assumed constant).
All three are taken as constants with respect to time.
Challenge! If 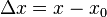 and
and 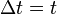 , derive:
, derive:
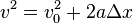 , and
, and 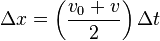
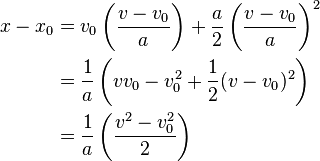
Solve 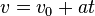 to obtain,
to obtain,
Insert this into
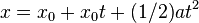 to obtain,
to obtain,Therefore,
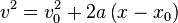 . Also,
. Also, 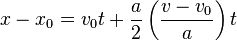 yields
yields 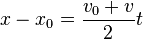 .
. Does this mean that the average velocity,
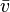 equals
equals 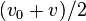 ? Yes, but only if the acceleration is uniform.
? Yes, but only if the acceleration is uniform.
Free-fall occurs when gravity is the only force that acts. The vector acceleration is
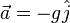 , where
, where 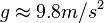 is the acceleration of gravity at Earth's surface.
The particle is at,
is the acceleration of gravity at Earth's surface.
The particle is at, 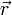

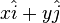 , and the velocity vector,
, and the velocity vector,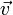 , is usually written in component form as,
, is usually written in component form as,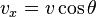 , and
, and 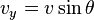 . The trajectory of free fall can be obtained from the initial conditions,
. The trajectory of free fall can be obtained from the initial conditions,
Render these equations for free-fall in component form and make a sketch illustrating the x and y components of 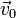 .
.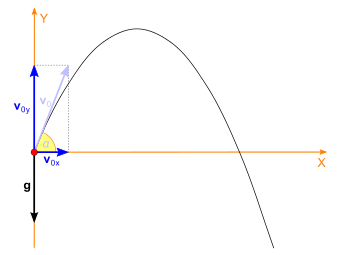 Components of initial velocity of parabolic motion
Components of initial velocity of parabolic motion
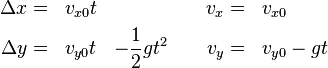
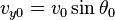 .
.