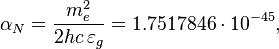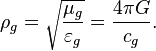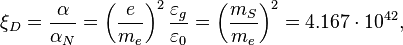Physics/Essays/Fedosin/Natural scale
< Physics < Essays < FedosinIn physics, Natural scale is the scale, which connects with the mass of electron. The method of constructing of the scale is the same as for Planck scale and Stoney scale. Usually, the Natural scale now considered for definition of the gravitational force between electrons and has not appropriate attention that should be for the scale of matter.
The natural gravitational coupling constant is:
where
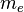 is the electron mass;
is the electron mass;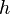 is the Planck constant;
is the Planck constant; is the speed of light in vacuum;
is the speed of light in vacuum;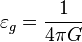 is the gravitoelectric gravitational constant;
is the gravitoelectric gravitational constant;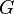 is the gravitational constant.
is the gravitational constant.
Fundamental units of vacuum
The set of primary vacuum constants is: [1] the speed of light  ; the vacuum permittivity
; the vacuum permittivity 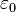 ; the speed of gravity
; the speed of gravity 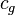 (usually equated to the speed of light); the gravitational constant
(usually equated to the speed of light); the gravitational constant 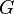 .
.
The set of secondary vacuum constants is:
The vacuum permeability: 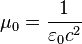 ;
;
The electromagnetic impedance of free space:
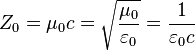 ;
;
The gravitoelectric gravitational constant:
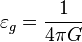 ;
;
The gravitomagnetic gravitational constant:
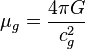 ;
;
The gravitational characteristic impedance of free space:
Natural scale units based on electron mass
For the sake of completeness in the Table 1 the main Natural scale units in the form consistent with Tables for the Stoney scale and vacuum constants are presented.
| Name | Dimension | Expressions | SI equivalent |
|---|---|---|---|
| Electron mass | Mass (M) | 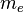 |
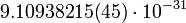 kg kg |
| Electron wavelength | Length (L) | 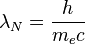 |
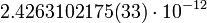 m m |
| Natural gravitational coupling constant | Dimensionless | 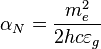 |
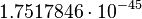 |
| Natural fictitious gravitational torsion mass | Dynamic mass (L2T −1) | 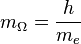 |
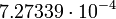 m2 s−1 m2 s−1 |
| Natural torsion coupling constant | Dimensionless | 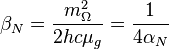 |
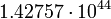 |
| Natural charge | Electric charge (Q) | 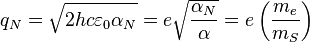 |
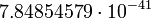 C C |
| Natural electric coupling constant | Dimensionless | 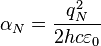 |
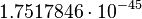 |
| Natural fictitious magnetic charge | Magnetic charge (L2MT −1Q−1) | 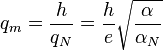 |
{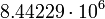 Wb Wb |
| Natural magnetic coupling constant | Dimensionless | 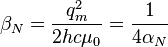 |
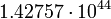 |
| Natural gravitational impedance quantum | Gravitational impedance (L2M−1T −1) | 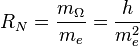 |
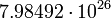 m2 kg−1 s−1 m2 kg−1 s−1 |
| Natural electromagnetic impedance quantum | Electrical impedance (L2M−1T −1Q−2) | 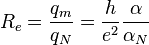 |
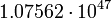 Ohm. Ohm. |
The difference of Natural coupling constant 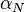 and fine structure constant
and fine structure constant 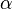 is so high, that the Natural scale now is the base for the Dirac large numbers and different numerology approaches. [2]
is so high, that the Natural scale now is the base for the Dirac large numbers and different numerology approaches. [2]
Actually, the relationship between fine structure constant and Natural coupling constant yields the Dirac number:
where 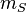 is the Stoney mass.
is the Stoney mass.
See also
- Planck scale
- Stoney scale
- Selfconsistent gravitational constants
- Selfconsistent electromagnetic constants
- Maxwell-like gravitational equations
- Quantum Gravitational Resonator