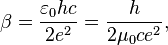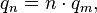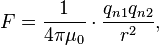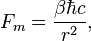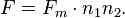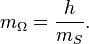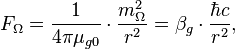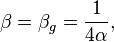Physics/Essays/Fedosin/Magnetic coupling constant

< Physics < Essays < Fedosin

In physics, the magnetic coupling constant, or magnetic fine structure constant (usually denoted β, the Greek letter beta) is a fundamental physical constant, characterizing the strength of the magnetic force interaction. The numerical value of β is the same in all system of measurement, because β is a dimensionless quantity: 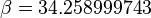$\beta = 34.258999743$ . The constant β was proposed by Yakymakha in 1989. ^[1]

Definition

The standard definition of the magnetic coupling constant is:

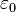\beta = \frac {\varepsilon_0 h c}{2 e^2} = \frac {h}{2 \mu_0 c e^2},

where:

$~ \varepsilon_0$ is the vacuum permittivity;
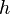$~h$ is the Planck constant;
$~c$ is the speed of light in vacuum;
$~ e$ is the elementary charge;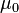
$~\mu_0$ is the vacuum permeability.

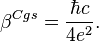
In the Cgs units magnetic coupling constant is: $\beta^{Cgs} = \frac{\hbar c}{4e^2} .\$

Physical interpretations

Magnetic charge quantization

It is known, that magnetic charge (and magnetic flux) has the property to be quantized:

q_n = n \cdot q_m, \

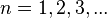where $n = 1,2,3,...$ is the integer number and 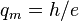$q_m =h/e$ is the fictitious elementary magnetic charge. The conception of magnetic monopole was first hypothesized by Pierre Curie in 1894,^[2] but the quantum theory of magnetic charge started with a 1931 paper by Paul Dirac. ^[3] In this paper, Dirac showed that the existence of magnetic monopoles was consistent with Maxwell's equations only in case of charge quantization, which is observed.

Magnetic force

Coulomb law for the fictitious magnetic charges is:

F = \frac{1}{4\pi \mu_0}\cdot \frac{q_{n1} q_{n2} }{r^2}, \

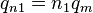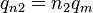where $q_{n1} = n_1 q_m$ and $q_{n2} = n_2 q_m$ are two interacting magnetic charges. At 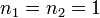$n_1 = n_2 = 1$ we shall have the minimal magnetic force:

F_m = \frac{\beta \hbar c}{r^2}, \

from which it is seen that 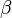$\beta$ is magnetic coupling constant .

In the general case, when we know the magnetic charges, Coulomb force could be rewritten as:

F = F_m\cdot n_1n_2. \

Gravitational torsion force

The "static" Stoney mass is defined as:

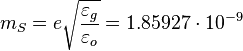m_S = e \sqrt{\frac{\varepsilon_g}{\varepsilon_o}} = 1.85927\cdot 10^{-9} \

kg,

where

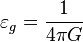\varepsilon_g = \frac{1}{4\pi G} \

is the gravitoelectric gravitational constant ,

G \

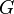is the gravitational constant.

Similar to the elementary magnetic charge the fictitious gravitational torsion mass could be defined:

m_{\Omega} = \frac{h}{m_S}. \

Newton law for the gravitational torsion masses is:

F_{\Omega } = \frac{1}{4\pi \mu_{g0}}\cdot \frac{ m_{\Omega}^2}{r^2} = \beta_g \cdot \frac{\hbar c}{r^2}, \

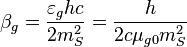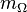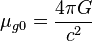where $\beta_g = \frac {\varepsilon_g h c}{2 m_S^2} = \frac {h}{2c \mu_{g0} m_S^2} \$ is the gravitational torsion coupling constant for the gravitational torsion mass $m_{\Omega } \$ , and $\mu_{g0} = \frac{4\pi G}{c^2} \$ is the gravitomagnetic gravitational constant.

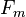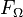In the case of equality of the above forces $F_m \$ and $F_{\Omega}\$ , we shall get the equality of the coupling constants for magnetic field and gravitational torsion field:

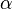\beta = \beta_g = \frac{1}{4 \alpha}, \

where $\alpha \$ is the fine structure constant as the coupling constant of electrostatic interaction.

From the stated above, it is evident that the magnetic coupling constant (magnetic fine structure constant) is the constant, which defines the force interactions (magnetic, gravitational, etc.) in the Stoney scale. Therefore, this dimensionless constant could be named as the Stoney scale dynamic force constant (with the following designation: 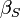$\beta_S \$ ).

References

↑ Yakymakha O.L.(1989). High Temperature Quantum Galvanomagnetic Effects in the Two- Dimensional Inversion Layers of MOSFET's (In Russian). Kyiv: Vyscha Shkola. p.91. ISBN 5-11-002309-3. djvu .
↑ Pierre Curie, Sur la possibilite d'existence de la conductibilite magnetique et du magnetisme libre (On the possible existence of magnetic conductivity and free magnetism), Seances de la Societe Francaise de Physique (Paris), p76 (1894). (fr)Free access online copy.
↑ Paul Dirac, "Quantised Singularities in the Electromagnetic Field". Proc. Roy. Soc. (London) A 133, 60 (1931). Free web link.

This article is issued from Wikiversity - version of the Tuesday, October 28, 2014. The text is available under the Creative Commons Attribution/Share Alike but additional terms may apply for the media files.