Introduction to group theory/Uniqueness of identity proof

Proof:

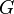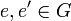Let $G$ be a group, and let $e,e' \in G$ both be identity elements. Then

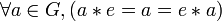\forall a \in G, (a*e=a=e*a)

and

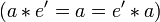(a*e'=a=e'*a)

Then since 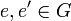$e,e' \in G$

e=e*e'=e'

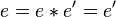and thus

e=e'

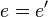Q.E.D.

This article is issued from Wikiversity - version of the Monday, March 26, 2012. The text is available under the Creative Commons Attribution/Share Alike but additional terms may apply for the media files.