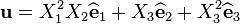Introduction to Elasticity/Sample quiz 3

< Introduction to Elasticity

Given the displacement vector

\mathbf{u} = X_1^2 X_2 \widehat{\mathbf{e}}_{1} + X_3 \widehat{\mathbf{e}}_{2} + X_3^2 \widehat{\mathbf{e}}_{3}
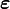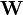  1. Find the strain tensor \boldsymbol{\varepsilon} and the rotation tensor \mathbf{W}\,.
  2. Write down the compatibility equation in the 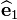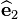\widehat{\mathbf{e}}_{1}- \widehat{\mathbf{e}}_{2} plane. Use index notation with commas representing partial derivatives with respect to Lagrangian material co-orinates.
This article is issued from Wikiversity - version of the Sunday, December 07, 2008. The text is available under the Creative Commons Attribution/Share Alike but additional terms may apply for the media files.