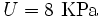Introduction to Elasticity/Sample midterm3
< Introduction to ElasticitySample Homework Problem 3
For an isotropic material with 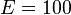 GPa and
GPa and 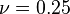 , find the stress tensor and strain energy density at a point in a body if the components of the strain tensor are given by
, find the stress tensor and strain energy density at a point in a body if the components of the strain tensor are given by
Solution
The shear modulus (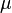 ) is given by
) is given by
The Lamé modulus (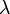 ) is given by
) is given by
The stress-strain relation for isotropic materials is
Therefore, (after converting 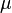 and
and 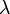 into KPa so that the
into KPa so that the
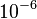 term in the strain cancels out),
term in the strain cancels out),
In 3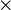 3 matrix form (after converting into MPa from KPa)
3 matrix form (after converting into MPa from KPa)
The strain energy density is given by
Therefore,
The strain energy density is
This article is issued from Wikiversity - version of the Tuesday, August 07, 2007. The text is available under the Creative Commons Attribution/Share Alike but additional terms may apply for the media files.
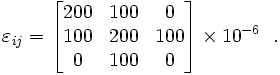
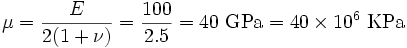
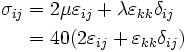
![\begin{align}
\sigma_{11} & = 40(2\varepsilon_{11} + \varepsilon_{11} + \varepsilon_{22}
+ \varepsilon_{33}) \\
& = 40\left[(3)(200) + 200 + 0\right] = (40)(800) = 32000 \\
\sigma_{22} & = 40(2\varepsilon_{22} + \varepsilon_{11} + \varepsilon_{22}
+ \varepsilon_{33}) \\
& = 40\left[(3)(200) + 200 + 0\right] = (40)(800) = 32000 \\
\sigma_{33} & = 40(2\varepsilon_{33} + \varepsilon_{11} + \varepsilon_{22}
+ \varepsilon_{33}) \\
& = 40\left[(3)(0) + 200 + 200\right] = (40)(400) = 16000 \\
\sigma_{23} & = 40(2\varepsilon_{23}) = (40)(200) = 8000\\
\sigma_{31} & = 40(2\varepsilon_{31}) = (40)(0) = 0\\
\sigma_{12} & = 40(2\varepsilon_{12}) = (40)(200) = 8000
\end{align}](../I/m/bf963362b49ab9b5c07bdb980f53133a.png)
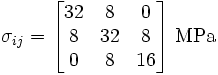
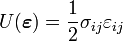
![\begin{align}
U(\boldsymbol{\varepsilon}) & = \frac{1}{2}\left[
\sigma_{11} \varepsilon_{11} +
\sigma_{22} \varepsilon_{22} +
\sigma_{33} \varepsilon_{33} +
2 \sigma_{23} \varepsilon_{23} +
2 \sigma_{31} \varepsilon_{31} +
2 \sigma_{12} \varepsilon_{12}
\right] \\
& = \frac{1}{2}\left[(32)(200) + (32)(200) + (16)(0) + (2)(8)(100) +
(2)(0)(0) + (2)(8)(100)\right]~\text{Pa}\\
& = \frac{1}{2}\left[6400 + 6400 + 1600 + 1600\right]~\text{Pa}\\
& = 8000~\text{Pa} = 8 ~\text{KPa}
\end{align}](../I/m/92bdbbecefc6e69a3d88a206d2379867.png)