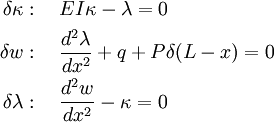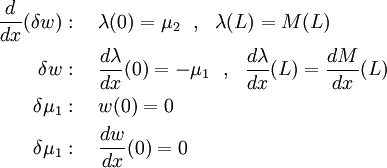Introduction to Elasticity/Energy methods example 4
< Introduction to ElasticityExample 4 : Bending of a cantilevered beam
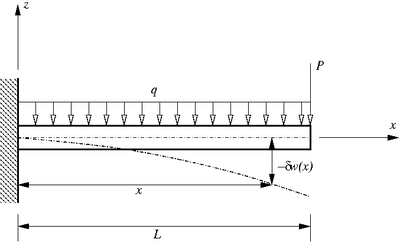 Bending of a cantilevered beam |
Application of the Principle of Virtual Work
The virtual work done by the external applied forces in moving through the virtual displacement 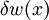 is given by
is given by
The work done by the internal forces are,
From beam theory, the displacement field at a point in the beam is given by
The strains are, neglecting Poisson effects,
and the corresponding stresses are
If we also neglect the shear strains and stresses, we get
Therefore, from the principle of virtual work,
Integrating by parts and after some manipulation, we get,
where 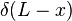 is the Dirac delta function,
is the Dirac delta function,
The Euler equation for the beam is, therefore,
and the boundary conditions are
Application of the Hellinger-Prange-Reissner variational principle
The governing equations of the cantilever beam can be written as
Kinematics
Constitutive Equation
Equilibrium (kinetics)
Recall that the Hellinger-Prange-Reissner functional is given by
If we apply the strain-displacement constraints using the Lagrange multipliers 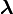 and the displacement boundary conditions using the Lagrange multipliers
and the displacement boundary conditions using the Lagrange multipliers 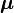 , we get a modified functional
, we get a modified functional
For the cantilevered beam, the above functional becomes
Taking the first variation of the functional, we can easily derive the Euler equations and the associated BCs.
and
The same process can be used to derive Euler equations using the Hu-Washizu variational principle.
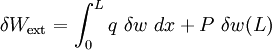
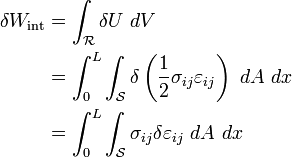
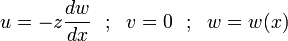
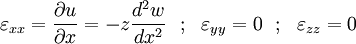
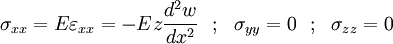
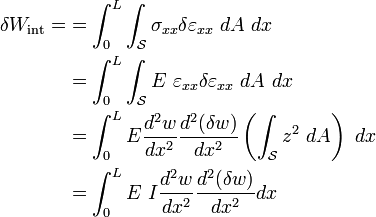
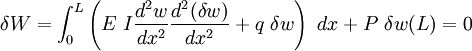
![\begin{align}
0 &= \int_0^L \left[\frac{d^2}{dx^2}\left(E~I\frac{d^2w}{dx^2}\right)
+ q + P\delta(L-x)\right]\delta w~dx +
\left.\left[\left(E~I\frac{d^2w}{dx^2}\right)\delta\left(\frac{dw}{dx}\right)
+ \frac{d}{dx}\left(E~I\frac{d^2w}{dx^2}\right)\delta w\right]\right|_0^L
\end{align}](../I/m/5dfa4e2dca8aebcd8fa6b6b69b5a2bbf.png)
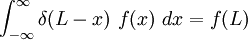
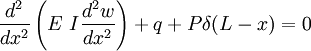
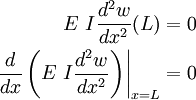
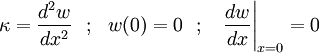
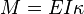
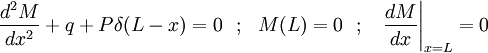
![{\mathcal H}[s] = \int_{\mathcal{B}} U^c(\boldsymbol{\sigma}) - \int_{\mathcal{B}} \boldsymbol{\sigma}:\boldsymbol{\varepsilon}~dV
- \int_{\mathcal{B}} \mathbf{f}\bullet\mathbf{u}~dV
+ \int_{\partial{\mathcal{B}}^{u}} \mathbf{t}\bullet(\mathbf{u}-\widehat{\mathbf{u}})~dA
+ \int_{\partial{\mathcal{B}}^{t}} \widehat{\mathbf{t}}\bullet\mathbf{u}~dA](../I/m/eb12330afbc99123c4c3a3907f8960f4.png)
![\bar{\mathcal H}[\mathbf{u},\boldsymbol{\varepsilon},\boldsymbol{\lambda},\boldsymbol{\mu}] = \int_{\mathcal{B}} \left[U(\boldsymbol{\varepsilon})
+ \boldsymbol{\lambda}:[\frac{1}{2}(\boldsymbol{\nabla}\mathbf{u}-\boldsymbol{\nabla}\mathbf{u}^T) - \boldsymbol{\varepsilon}]
- \mathbf{f}\bullet\mathbf{u}\right]~dV
- \int_{\partial{\mathcal{B}}^{u}} \boldsymbol{\mu}\bullet(\mathbf{u}-\widehat{\mathbf{u}})~dA
- \int_{\partial{\mathcal{B}}^{t}} \widehat{\mathbf{t}}\bullet\mathbf{u}~dA](../I/m/270a0d9457a5d22b5949908d334a5559.png)
![\begin{align}
\bar{\mathcal H}[w,\kappa,\lambda,\mu_1,\mu_2] = &
\int_0^L \left[\frac{EI}{2}\kappa^2
+ \left(\frac{d^2w}{dx^2}-\kappa\right)\lambda
+ [q + P\delta(L-x)]~w\right]~dx \\
& - M(L)\frac{dw}{dx}(L) - \frac{dM}{dx}(L)~w(L)
+ \mu_1[w(0) - 0] + \mu_2[\frac{dw}{dx}(0) - 0]
\end{align}](../I/m/89e7547a1164e433fbf046ea5b0b3cee.png)