Fourier analysis

Introduction

Fourier analysis is a method of analysing functions. These functions may be electrical signals (say, from an electronic circuit being tested), pure mathematical functions, or any kind of data being analysed on a computer. Regardless, if the function is single-valued, Fourier analysis can be used to produce an imperfect approximation.

The trigonometric form

Fourier analysis works by breaking down the function being considered into a Fourier Series. The Fourier Series, in simplest terms, is a summation of sine and cosine functions. Each of these trigonometric functions looks something like this:

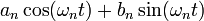
The exponential form

Consider f(x) as real valued function

f(x)=ΣA_n e^{inx [1]}