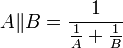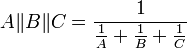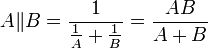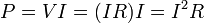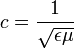Electricity/Introduction

< Electricity

Engineering

Resource type: this resource is a course.

Subject classification: this is an engineering resource .

Educational level: this is a secondary education resource.

Educational level: this is a tertiary (university) resource.

Let us begin with a few fundamental ideas about electricity to anchor all of your future lessons...

Fundamental Background

First of all, you might ask yourself, 'what exactly is electricity?'

At the level of atomic physics, electricity is one of the fundamental forces of nature, arising from an "electric charge" possessed by subatomic particles (notably protons and electrons).

At a practical level, electricity describes the phenomena associated with the bulk behavior of aggregates of enormous numbers of those charged particles in normal matter. Just as the observed mass of ordinary objects arises from the mass of the constituent particles, the observed behavior of electricity in ordinary objects arises from the electric charges of the constituent subatomic particles. In fact, ordinary electrical phenomena arise from incredibly tiny differences between the number of positively charged protons and negatively charged electrons. In ordinary "electrically neutral" matter, the positive and negative charges cancel essentially exactly, so no electrical properties are observed. The imbalance between protons and electrons in matter exhibiting electrical phenomena is exceedingly small—a golf ball with a charge of 10,000 volts has an electron excess or deficit of only 1 part in 10¹³.

Electricity in Practice

The preceding description in terms of fundamental particles and forces is perhaps too theoretical for practical use. What is Electricity in practice?

The tiny imbalance of positive and negative charges discussed above is caused by electrons moving (in enormous numbers at the atomic scale but very tiny numbers percentage-wise) away from the atomic nuclei to which they belong. This motion is perhaps best visualized as a fluid. The hydraulic concepts of fluid flow, pressure, and friction make a good analogy.

In fact, early researchers in electricity in the 18^th and 19^th centruries saw this, and referred to the "electrical fluid". They even noticed that this "fluid" could be stored in "Leyden jars", which were glass jars with metal foil on the inside and outside. We now know that the jars were just capacitors with the glass as the dielectric, and that the fact that they were jars was a coincidence. We now rate capacitors in farads; early researchers rated them in quarts.

The unit of quantity of electric charge is the coulomb, abbreviated "C", and named after Charles-Augustin Coulomb.^[1] It is 6.241 x 10¹⁸ elementary charges. Or equivalently, the elementary charge (charge on a single electron or proton) is 1.602 x 10^-19 coulombs. In the hydraulic analogy, it is analogous to a liter of fluid. But unlike water, electric charge can be either positive (deficit of electrons) or negative (excess of electrons.)

Flow of charge—what we call current—could be thought of as flow of positive charge in one direction, or of negative charge in the other direction. By convention, current flow is always considered to be the flow of positive charge, even though that is contrary to the actual flow of (negative) electrons. Hence the current is considered to flow out of the positive terminal of a battery, through whatever is being powered, and back into the negative terminal, even though electron flow through the wires is in the opposite direction.

The unit of current flow is the ampere, abbreviated "A", which is a flow rate of one coulomb per second. It is named after André-Marie Ampère. In the hydraulic analogy, it is analogous to the amount of flow through a pipe, or over a dam, etc., which might be measured in liters per second with the hydraulic analogy.

Energy, Power, and Voltage

We will continue to use the hydraulic analogy. It takes energy to pump water uphill, and the potential energy of being at a high elevation is released when it descends to a lower elevation (The Siphon Principle). The amount of energy (in joules) is 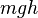$mgh$ , where 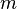$m$ is the mass of liquid (say, in kilograms), 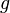$g$ is the strength of gravity (9.8 meters per second squared, or 9.8 newtons per kilogram), and 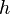$h$ is the elevation distance.

Some elementary physics: The SI unit of energy is the joule, and is one newton-meter, and is the energy required to push something with a force of one newton through a distance of one meter. The unit of force is the newton, and is the force required to increase the speed of a one kilogram object by one meter per second per second. The unit of rate of flow of energy is the watt, and is one joule per second. Note that the watt relates to all of physics, not just electricity.

For a given difference in elevation 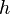$h$ , it takes 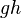$gh$ joules of energy per kilogram to lift water (or any other liquid) through that height. That same amount of energy is released when the water flows down by that height. It might drop directly—old-fashioned water wheels use this method, or the water might be stored in a reservoir, causing the water to have enormous pressure at the bottom. That pressure gives the water a lot of energy per kilogram, which can be recovered by turbines—modern hydroelectric power stations do this. The amount of pressure at the bottom of a column of water of height 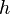$h$ is enough to release 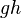$gh$ joules per kilogram.

The electrical analogue of this pressure is voltage. The unit is the volt, named after Alessandro Volta. It is the amount of "pressure" that releases one joule per coulomb of charge. A given region of space (or, more commonly in practice, a given point in an electrical circuit) has a certain voltage potential. If two points differ in potential by V volts, then the passage of Q coulombs of charge from one point to the other releases QV joules of energy. This "potential difference" or "voltage difference" is analogous to the difference in height (and hence difference in pressure) between water at the top of a reservoir and water at the bottom.

In fact, some very old books refer to voltage as "electrical pressure". Another term for voltage that one sees in older books is "electro-motive force" or its abbreviation "emf". It's just voltage.

Since the release of one joule per second of energy is a release of one watt of power, a flow of one coulomb per second, that is, a flow of one ampere, across a voltage difference of one volt releases one joule per second, that is, one watt of power.

power (W, that is, watts) = voltage (V, that is, volts) times current (A, that is, amperes)

There is another useful analogy to water. The level of water on a pond is uniform, that is, the surface is level. This is because the water can travel very easily from one point to another. If the surface were higher at one point than at another, the water would simply flow from the higher point to the lower one, until equilibrium is reached. Similarly, in a conductor (e.g. a wire), electrons can flow very easily from one point to another, so there is essentially no voltage difference. If there were, the electrons in the wire would simply flow from the higher voltage to the lower voltage (well, actually, they would flow the other way because their charge is negative) until the voltage is equalized. Hence, all points in an electric circuit that are connected by a wire are at the same voltage.

This only applies to situations in which the water or the charges can move easily. In a river, there is some resistance to the motion, so the water is constantly moving from higher elevations to lower elevations. Not all conductors are perfect, so there is actually a slight voltage drop (and dissipation of power) across a conductor. How great this effect is depends on the resistance, in accordance with Ohm's law, which we will discuss presently.

Symbols and Abbreviations

The symbols used for quantities in electrical engineering can be confusing, since the symbol for a quantity may be different from the symbol for the units in which it is measured. We list the common symbols here, even though we have not yet defined all of the concepts involved.

Electrical charge is typically denoted 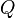$Q$ , measured in coulombs, abbreviated 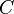$C$ . However, electrical engineers rarely refer to charge, and the letter 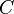$C$ is usually used to denote capacitance.

Example:

Q = CV\,

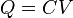is the theoretical formula for a capacitor's behavior—the charge is the product of the capacitance times the voltage.

Current is typically denoted 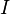$I$ , measured in amperes, abbreviated $A$ .

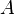An ampere is, among other things, a coulomb per second.

Example:

V = IR\,

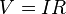Ohm's law; voltage equals current times resistance.

Example: "

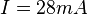I = 28mA\,

" "The current is 28 milliamperes."

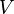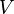Voltage is typically denoted $V$ (or sometimes $E$ ), and measured in volts, abbreviated $V$ . The use of " $E$ " stands for "emf" (electro-motive force.)

A volt is, among other things, a joule per coulomb.

Example: "

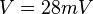V = 28mV\,

" "The voltage is 28 millivolts."

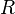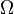Resistance is typically denoted $R$ , measured in ohms, abbreviated with the capital Greek omega: $\Omega$ .

An ohm is, among other things, a volt per ampere.

Example: "

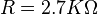R = 2.7K\Omega\,

" "The resistance is 2.7 kilohms."

It is sometimes useful to speak of the reciprocal of resistance. This is called conductance, and is typically denoted 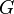$G$ , traditionally measured in "mhos" ("mho" is "ohm" spelled backwards), abbreviated with an upside-down omega: 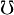$\mho$ . A less flippant term than "mho" has been adopted: the siemens, abbreviated $S$ .

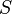A mho/siemens is, among other things, an ampere per volt.

Example:

I = VG\,

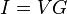Ohm's law rewritten in terms of conductance.

Example: "

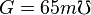G = 65m\mho\,

" "The conductance is 65 millimhos."

Example: "

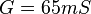G = 65mS\,

" "The conductance is 65 millisiemens."

Capacitance is typically denoted 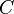$C$ , measured in farads, abbreviated $f$ .

A farad is, among other things, a second per ohm, or a coulomb per volt.

Example:

t = RC\,

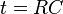The time constant is the resistance times the capacitance.

Example: "

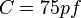C = 75pf\,

" "The capacitance is 75 picofarads."

Inductance is typically denoted 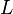$L$ , measured in henries, abbreviated $h$ .

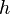A henry is, among other things, an ohm-second.

Example:

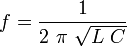f = \frac{1}{2\ \pi\ \sqrt{L\ C}}

is the formula for the frequency of a resonant circuit.

Example: "

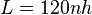L = 120nh\,

" "The inductance is 120 nanohenries."

Power is typically denoted 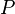$P$ , measured in watts, abbreviated $W$ .

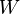A watt is, among other things, a joule per second, or a volt-ampere.

Example:

P = VI\,

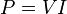The power is the voltage times the current.

Example: "

P = 75W\,

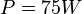" "The power is 75 watts."

The frequency of an oscillation or signal is typically denoted $f$ , measured in hertz, abbreviated $Hz$ .

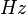A hertz is really just a reciprocal second. In fact, the unit of frequency used to be just "cycles per second" or simply "cycles".

Example: "

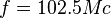f = 102.5 Mc\,

" "102.5 megacycles on the FM dial" (old way.)

Example: "

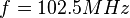f = 102.5 MHz\,

" "102.5 megahertz on the FM dial" (new way.)

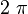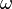It happens that, in a lot of the mathematical formulas the unit of radians per second is superior. A frequency in radians per second is $2\ \pi$ times the frequency in hertz. When measured this way, the symbol $f$ is replaced with the lower-case Greek omega: $\omega$ . Many occurrences of 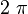$2\ \pi$ disappear from various formulas when radians are used.

Example:

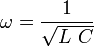\omega = \frac{1}{\sqrt{L\ C}}

is the formula for the frequency of a resonant circuit, in radians per second.

Example:

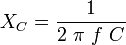X_C = \frac{1}{2\ \pi\ f\ C}

is the formula for capacitive reactance, calibrated in hertz.

Example:

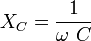X_C = \frac{1}{\omega\ C}

is the formula for capacitive reactance, calibrated in radians per second.

Example: "

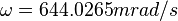\omega = 644.0265mrad/s\,

" "644.0265 megaradians per second on the FM dial" (not known to have ever been announced.)

Static Electricity and "Current" Electricity

Electricity manifests itself in two seemingly different ways. They are different manifestations of the same thing. "Static" electricity was known to the ancients. It involves very high voltages and very low currents—the currents are so low that one doesn't always realize how high the voltage is. In order for such high voltages to persist somewhere, the insulation must be extremely good, that is, the resistances must be extremely high. Fortunately for the history of science, materials like glass, amber, and some types of rubber and other materials, have the necessary high resistance. (The word "electricity" comes from the Greek word for amber.)

The voltages involved with static electricity are high enough to make the leaves of an electroscope move, but the currents are so low that we would normally not notice any effect of the current. "Current" electricity involves lower voltages and currents large enough to power light bulbs, motors, and such. Rubbing a glass rod with a piece of silk can't come anywhere near to providing the required level of sustained current. To get the required sustained levels of current requires either ongoing chemical reactions (as in a battery) or electromechanical devices (as in a generator.) The ability to do this was discovered in the 18^th and 19^th centuries by Luigi Galvani, Alessandro Volta and Michael Faraday.

The study of electronics and electrical engineering involve "current" electricity almost exclusively.

Series and Parallel Connections

Schematic diagram showing series and parallel connections.

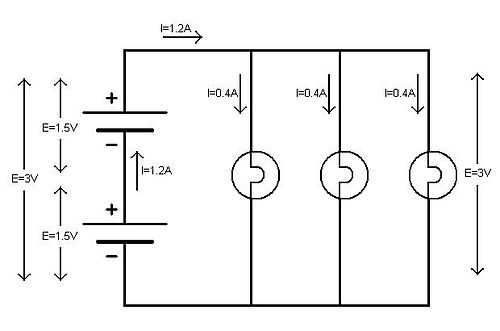
If we were to stack three hydroelectric dams on top of each other (that is, make the reservoir three times as deep), the pressure at the bottom would be three times as great, and the energy obtained per liter of water would be three times as great. (Or, equivalently, we make an old-fashioned water wheel with three times the vertical drop.) The electrical equivalent of this is the series connection, or the placement of electrical components in series. The voltage difference across components connected in series is the sum of the voltage differences across the individual components, but the current is the same through each component.

If we were to place three hydroelectric dams next to each other (that is, just triple the flow of water), the pressure would be the same, so the amount of energy per liter would be the same, but the overall flow of water would be three times as great, so the total power would be three times as great. The electrical equivalent of this is the parallel connection, or the placement of electrical components in parallel. The total current through components connected in parallel is the sum of the currents through the individual components, but the voltage difference is the same.

The diagram at the right shows a simple flashlight, with two batteries connected in series and three light bulbs connected in parallel. The total voltage across the pair of batteries is 3 volts, and the current through each is 1.2 amperes. The light bulbs are connected in parallel. The voltage across each is 3 volts, and the current through each is 0.4 amperes. Each battery provides 1.8 watts of power (1.5 volts times 1.2 amperes), so both batteries combined provide 3.6 watts. Each light bulb has 0.4 amperes flowing through it, so it consumes 1.2 watts. The three bulbs in combination consume 3.6 watts.

This is an example of a schematic diagram—an abstract diagram showing how electrical components are interconnected. You could not possibly build a satisfactory house, automobile, or telescope from such an abstract description ("build roof; place on top of walls"). But electricity, passing through wires from one component to another, is so well behaved that, for ordinary electrical projects, a schematic diagram is all you need. Schematic diagrams ("schematics") are the principal workhorse of electrical engineering.

The terms "series" and "parallel" are actually used quite loosely by electrical engineers, to describe aspects of circuit topology. For example, the flashlight depicted above lacks an on-off switch. One would put the switch in series with the parallel assemblage of light bulbs.

Ohm's Law

We use the hydraulic analogy one last time. If we increase the pressure of water going into a pipe, the flow will increase. The flow will be roughly proportional to the pressure. For wider pipes, the proportionality constant will be different than for narrower pipes.

There is a similar principle for electricity: Ohm's law, discovered by Georg Simon Ohm in the 1820's, states that the current through a resistive medium is proportional to the applied voltage. Whereas the linearity of water flow and pressure is only approximate, Ohm's law is extremely accurate for virtually all substances of interest, other than things that make use of special semiconductor properties. The proportionality constant, for a given device, is the resistance, measured in ohms. Ohm's law is:

V = IR\,

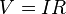Voltage equals current times resistance.

Also stated, of course, as:

I = V/R\,

R = V/I\,

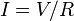
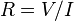
Simple analysis of series and parallel connections (a long wire is equivalent to two shorter wires in series; a thick wire is equivalent to two thinner wires in parallel) shows that the resistance of an object made of some substance is proportional to the length and inversely proportional to the cross-sectional area. One can therefore see that the proper measure of the resistivity of a substance is "ohm meters". This is a somewhat non-intuitive way of expressing something, and a more common way is to measure the conductivity, which is the reciprocal of resistance, in siemens per meter.

Electrical resistivity varies by over 20 orders of magnitude between metals and air, and over 30 orders of magnitude between metals and various insulating materials. This is why it is possible to send telephone and other cable communications through many miles of wire, where the wire thickness is a fraction of a millimeter, with virtually no leakage between adjacent wires.

Here are a few approximate conductivities, in siemens per meter:

Superconductors: infinity (because of complicated quantum-mechanical phenomena)
Silver: 6 * 10⁷
Silicon: 2 * 10^-3 (but, in semiconductor materials, it is "doped" with impurities, giving it a much higher conductivity)
Glass: about 10^-12
Air: about 10^-14
Paraffin: about 10^-17
Teflon: about 10^-23

Electrical devices that are intended to exhibit resistance are resistors. They are manufactured in resistances from a small fraction of an ohm to many megohms.

From what we know about ohm's law, and about voltages and currents in series and parallel connections, we can derive the rules for resistors in series and parallel:

When resistances of

A\,

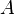and

B\,

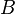are placed in series, the resultant resistance is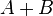

A+B\,

When resistances of

A\,

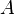and

B\,

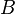are placed in parallel, the resultant resistance is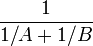

\frac{1}{1/A+1/B}\,

The latter is known as the "reciprocal of the sum the reciprocals" law. A special mathematical symbol for this operation is sometimes used: the "parallel" symbol from elementary geometry:

A\|B = \frac{1}{\frac{1}{A} + \frac{1}{B}}

A\|B\|C = \frac{1}{\frac{1}{A} + \frac{1}{B} + \frac{1}{C}}

This operation is so useful that one sometimes sees this symbol in scientific and mathematical contexts not related to electricity.

There is a quick "cheat" for this operation, when there are just two resistors—the product divided by the sum but it only works for two resistors:

A\|B = \frac{1}{\frac{1}{A} + \frac{1}{B}} = \frac{A B}{A + B}

"I-squared-R" and Joule's law

One sometimes encounters phrases like "I-squared-R losses" in a wire. This is a combination of the power formula and Ohm's law.

P = VI = (IR) I = I^2 R\,

Notice that the amount of power lost in a wire is proportional to the square of the current.

The phenomenon of heat being evolved when electricity passes through a conductor is sometimes called Joule's law. It's really just a consequence of conservation of energy and the equivalence of heat to other forms of energy.

Kirchoff's Laws

There are two famous "laws" of electrical engineering, known as Kirchhoff's Voltage Law and Kirchhoff's Current Law. They are really just straightforward applications of what has been discussed above.

Kirchhoff's Voltage Law (sometimes abbreviated KVL): The sum of the voltage drops around any closed path in a circuit is zero. This is just conservation of energy—a quantity of electric charge could pick up energy for free by going around a closed loop with a nonzero total voltage drop. Remember that "voltage drop" between two points can be either positive or negative.

Kirchhoff's Current Law (sometimes abbreviated KCL): The sum of the currents flowing into a point in a circuit is zero. This is just conservation of charge. Remember that current flow "into" a point is negative if the current is flowing out. now

How Are the Units Defined?

As one can tell from the connections among the electrical units and the physical units such as newtons, joules, and watts, a lot of care went into the design of the system of units. But how is the coulomb defined? Why is it equal to 6.241 x 10¹⁸ elementary charges?

The coulomb and volt are defined in terms of the ampere, so that a volt times an ampere equals a watt, that is, a newton-meter per second. And a coulomb is an ampere-second.

The ampere was determined experimentally, as follows: it is the amount of current flowing in each of two infinitely long and infinitely thin parallel wires separated by a distance of one meter that causes an attractive magnetic force of 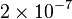$2 \times 10^{-7}$ newtons per meter along the length of the wires. Why the factor of 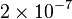$2 \times 10^{-7}$ ? This was chosen to make the volt a reasonable quantity relative to the voltage coming out of batteries.

This definition of the units in terms of the magnetic force has the effect of defining the fundamental constant of the magnetic force, labeled 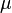$\mu\,$ , to be 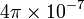$4 \pi \times 10^{-7}\,$ . The fundamental constant of the electric force, labeled $\epsilon\,$ , is related to this, according to the formula

c = \frac{1}{\sqrt{\epsilon \mu}}\,

where $c\,$ , the speed of light, was traditionally determined experimentally. ^[2]

The Next Lecture

For the next lecture, see Introduction to Electricity II.

Footnotes and References

↑ There are an enormous number of electrical units, and an enormous number of scientists after whom they are named—Coulomb, Volt, Ampere, Farad, Henry, Gauss, Weber, Tesla, Gilbert, Oersted, Hertz, Siemens, and others.
↑ The constant $c\,$ , the speed of light, is now defined to be 299,792,458 meters per second. A second is officially defined in terms of a cesium clock and the meter is derived from that.

Electricity/Introduction

Fundamental Background

Electricity in Practice

Energy, Power, and Voltage

Symbols and Abbreviations

Static Electricity and "Current" Electricity

Series and Parallel Connections

Ohm's Law

"I-squared-R" and Joule's law

Kirchoff's Laws

How Are the Units Defined?

The Next Lecture

Footnotes and References

See Also