Derivatives
Derivative of a function  at a number
at a number 
Notation
We denote the derivative of a function  at a number
at a number  as
as 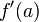 .
.
Definition
The derivative of a function  at a number
at a number  a is given by the following limit (if it exists):
a is given by the following limit (if it exists):
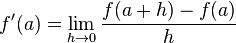
An analagous equation can be defined by letting 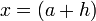 . Then
. Then 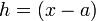 , which shows that when
, which shows that when 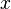 approaches
approaches  ,
, 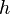 approaches
approaches 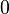 :
:
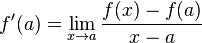
Interpretations
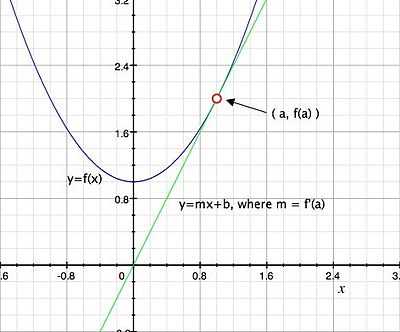
As the slope of a tangent line
Given a function 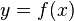 , the derivative
, the derivative 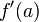 can be understood as the slope of the tangent line to
can be understood as the slope of the tangent line to 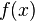 at
at  :
:
As a rate of change
The derivative of a function 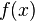 at a number
at a number  can be understood as the instantaneous rate of change of
can be understood as the instantaneous rate of change of 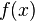 when
when  .
.
At a tangent to one point of a curve
Vocabulary
The point A(a ; f(a)) is the point in contact of the tangent and Cf.
Definition
If f is differentiable in a, then the curve C admits at a point A which has for coordinates (a ; f(a)), a tangent : it is the straight line passing by A and of direction coefficient f'(a). An equation of that tangent is written: y = f'(a)*(x-a)+f(a)
| Go to the School of Mathematics |