Boolean function

☞ This page belongs to resource collections on Logic and Inquiry.

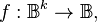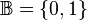A finitary boolean function is a function of the form $f : \mathbb{B}^k \to \mathbb{B},$ where $\mathbb{B} = \{ 0, 1 \}$ is a boolean domain and where 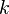$k\!$ is a nonnegative integer. In the case where 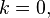$k = 0,\!$ the function is simply a constant element of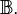 $\mathbb{B}.$

There are 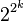$2^{2^k}$ such functions. These play a basic role in questions of complexity theory as well as the design of circuits and chips for digital computers.

Syllabus

Focal nodes

Peer nodes

Logical operators

Relational concepts

Information, Inquiry

Document history

Portions of the above article were adapted from the following sources under the GNU Free Documentation License, under other applicable licenses, or by permission of the copyright holders.

This article is issued from Wikiversity - version of the Thursday, November 05, 2015. The text is available under the Creative Commons Attribution/Share Alike but additional terms may apply for the media files.