Waves/Beats

< Waves

Waves : 1 Dimensional Waves
1 - 2 - 3 - 4 - 5 - 6 - 7 - 8 - 9 - 10 - 11 - 12 - 13
Examples - Problems - Solutions - Terminology

Beats

Suppose two sound waves of different frequency impinge on your ear at the same time. The displacement perceived by your ear is the superposition of these two waves, with time dependence 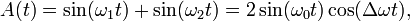$A(t) = \sin ( \omega_1 t) + \sin ( \omega_2 t) = 2 \sin ( \omega_0 t) \cos ( \Delta \omega t) ,$ (2.19)

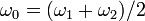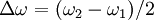where we now have $\omega_0 = ( \omega_1 + \omega_2 )/2$ and $\Delta \omega = ( \omega_2 - \omega_1 )/2$ . What you actually hear is a tone with angular frequency 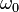$\omega_0$ which fades in and out with period 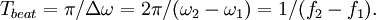$T_{beat} = \pi / \Delta \omega = 2 \pi / ( \omega_2 - \omega_1 ) = 1 / ( f_2 - f_1 ) .$ (2.20)

The beat frequency is simply 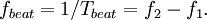$f_{beat} = 1/T_{beat} = f_2 - f_1 .$ (2.21)

Note how beats are the time analog of wave packets -- the mathematics are the same except that frequency replaces wavenumber and time replaces space.

Waves : 1 Dimensional Waves
1 - 2 - 3 - 4 - 5 - 6 - 7 - 8 - 9 - 10 - 11 - 12 - 13
Examples - Problems - Solutions - Terminology

This article is issued from Wikibooks. The text is licensed under Creative Commons - Attribution - Sharealike. Additional terms may apply for the media files.