Trigonometry/The Unit Circle

The Unit Circle is a circle with its center at the origin (0,0) and a radius of one unit.

Unit Circle

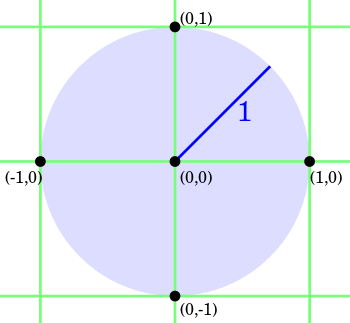
Angles are always measured from the positive x-axis (also called the "right horizon"). Angles measured counterclockwise have positive values; angles measured clockwise have negative values.

Defining Sine and Cosine in terms of the unit circle

In the unit circle shown here, a unit-length radius has been drawn from the origin to a point (x,y) on the circle.

Defining sine and cosine

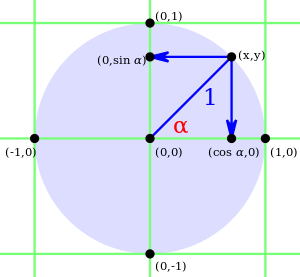
A line perpendicular to the x-axis, drawn through the point (x,y), intersects the x-axis at the point with the abscissa x. Similarly, a line perpendicular to the y-axis intersects the y-axis at the point with the ordinate y. The angle between the x-axis and the radius is $\alpha$ .

So, we can say that the sine of an angle is the ordinate of the point on the unit circle at that angle, and, the cosine of an angle is the abscissa of the point on the unit circle at that angle.

We define the basic trigonometric functions of any angle $\alpha$ as follows:

${\begin{matrix}\mathrm {Sine:} &\sin(\alpha )&=&y\\\mathrm {Cosine:} &\cos(\alpha )&=&x\\\end{matrix}}$

$\tan(\theta )$ can be algebraically defined.

$\tan(\theta )={\frac {\sin(\theta )}{\cos(\theta )}}\qquad \cos(\theta )\neq 0$

$\tan(\alpha )={\frac {y}{x}}\qquad x\neq 0$

These three trigonometric functions can be used whether the angle is measured in degrees or radians as long as it specified which, when calculating trigonometric functions from angles or vice versa.

Alternative definitions

A previous chapter used Soh-Cah-Toa to define the trigonometric functions. The advantage of the unit circle is that θ can be extended outside the first quadrant $\left[0,{\frac {\pi }{2}}\right]$ , which allows us to define these functions on the interval $(-\infty ,\infty )$ .
If trigonometry is applied to vectors, it is more convenient if the radius of the circle is not equal to unity. For example, if vector A has magnitude A=|A|:

${\begin{matrix}x=r\cos(\alpha )&\to &A_{x}=A\cos(\alpha )\\y=r\sin(\alpha )&\to &A_{y}=A\sin(\alpha )\\r^{2}=x^{2}+y^{2}&\to &A^{2}=A_{x}^{2}+A_{y}^{2}\\\end{matrix}}$

Video Links

More about this topic can be found at the 'Khan Academy:'

Some Values for Sine and Cosine

A unit circle with certain exact values marked on it is below:

Labeled Unit Circle

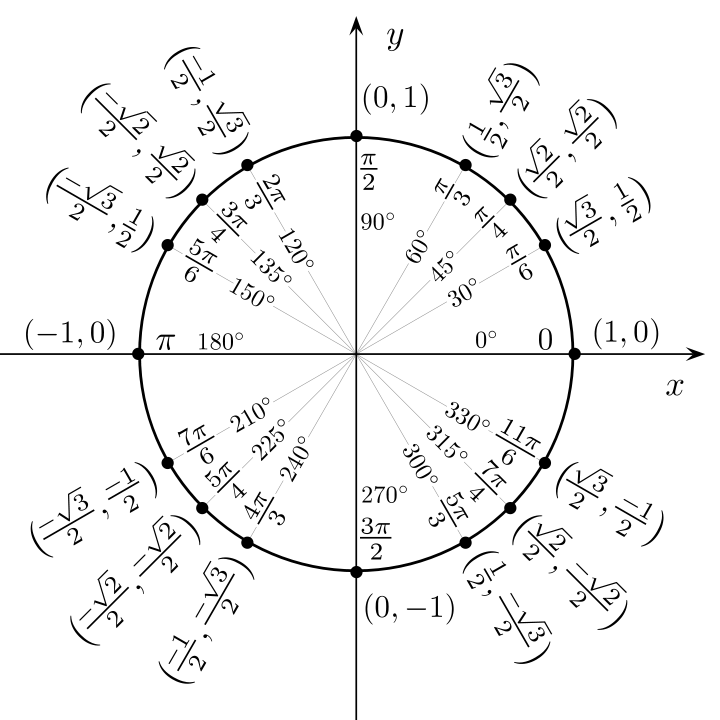
It is worth memorizing some of the values of sine and cosine on the unit circle (cosine is equal to x while sine is equal to y). You should at least become familiar with the values for $0^{\circ },30^{\circ }45^{\circ },90^{\circ }$ and know where ${\frac {\pi }{2}},\pi ,{\frac {3\pi }{2}},2\pi$ are on the unit circle.

Unit circles form the basis of most analog clocks and animations on computers since the cos and sin correspond to the x and y positions of the end of the line segments representing the hands of the clock.

Clock Hands

What angle (a) in degrees and (b) in radians do the hour and minute hands of a clock move through in:
One hour
One minute
How far apart in degrees are the hour and minute hand at five minutes past five? (you should take into account that the hour hand is not exactly at the five, but has moved a little further)

This article is issued from Wikibooks. The text is licensed under Creative Commons - Attribution - Sharealike. Additional terms may apply for the media files.