Trigonometry/Teachers Notes/About This Book
< Trigonometry < Teachers NotesThis page explains for someone who already knows trigonometry the structure and contents of the book(s). We also indicate the pedagogical points being made. This is to help teachers, but it also gives guidance when someone wants to add material as to where the material should go - without them having first to have read the whole book.
Features of the Books (at a Glance)
There are three books:
- Book 1, basic trig, is extensively cross referenced to Khan Academy Videos.
- Book 2 explores some of the prettier, but usually not examined extensions especially various circles relating to triangles.
- Book 3 relates sine cosine and exponential function, uses power series, complex numbers and calculus.
All three books:
- Have sections for enthusiasts
- Have both worked examples and exercises.
- Pages where maths is directly applied to Computer Science are marked with a symbol.
Some differences between this book and more traditional texts include a greater interest in "how do you check this?", "how do you learn/remember this?", "what conventions are arbitrary, and what is the reason for the ones which aren't arbitrary?", "why do we care at all about proof?".
Trigonometry Book 1
Basic Trigonometry This section is roughly at the same level as the Khan Academy Trigonometry course (K-12) and is designed to work well with it. We cannot assume that students are fluent with algebra, and so in this section we take many explanations step by step. We use the convention used in German schools of writing on the right hand side what we are about to do in the next step.
We work well with other resources, videos, on-line exercises e.t.c., and as a convenience provide links where relevant. We have our own exercises too. It is not by any means essential to have access to the external links to use our course. Generally our on-page exercises are more interesting than can be created automatically, which we hope will encourage students to actually do them, because of course it is essential that students practice the skills somehow and actually engage with the course.
Trigonometry Book 2
Geometric Extensions - not exam material (usually) This has more pre-calculus trigonometry, though using some vector and matrix maths. The algebra moves at a brisker pace than in Book 1 but vector and matrix maths is explained in detail where it is used. The topics are less central to understanding trigonometry than in Book 1. For many standard trigonometry courses Book 2 would be optional material. This book deepens the understanding of the many relationships between triangles and circles and uses trigonometric functions to investigate ellipses and spirals. It shows how to tackle some harder trigonometric function identities. Some of the geometric material is motivated by computer applications of trigonometry.
Trigonometry Book 3
Trig with Calculus and Complex Numbers For this more advanced section the reader will need to have done some basic calculus, to be comfortable with polynomials and differentiation and to 'get' the idea of the 'square root of minus one'. More fluency with algebra is assumed. That's a big jump from the basic sections, which is why we don't mix the three sections.
Some of the topics touch on deep mathematics. The point of doing this is that the way trigonometry leads into deeper water via the Fourier transform is deep. Bringing in some of the working tools of more advanced material, Pontryagin duality, Eigenfunctions, actually helps to make it all a lot clearer. One can see this by analogy. Addition formulae from trigonometry are a great deal simpler and less arbitrary from the high ground of complex analysis.
Sub-chapters in each 'For Enthusiasts'
All three books have chapters 'for enthusiasts'.
- An example in the Book 1 is calculating the distance between New York and Tokyo using their latitude and longitude and the radius of the Earth. That's clearly for enthusiasts. It is a more involved calculation than you'd normally expect someone to do at the basic level. The Basic for-enthusiasts section only uses the trigonometry that has been taught by that stage, no calculus, complex numbers or simultaneous equations.
- An example in Book 2 is Delaunay triangulation, which has many geometry related applications in computing. The main book 2 section on the Circumcircle introduces the necessary vector maths of triple product.
- An example in the Book 3 is Chebyshev polynomials, showing how (a) they are an expansion in terms of cosine/sine and (b) why they approach the min-max polynomials (for the interval [-1,1]).
Trigonometry Book 1 Contents
Does not include calculus, power series, vectors or complex numbers. Terms will ALWAYS be explained on the page where they are first used.
- Introduction We give a formula straight away on the front page (Pythagoras). We get across that formulas are central, this is maths, no point denying it, and say what trigonometry is for.
- Angles of a triangle sum to 180 Degrees: We use this page to get across the idea that you can calculate more information about a triangle given some of the information. Terminology 'isosceles', 'equilateral' and 'congruent' are introduced. We also start very early on forming the idea of 'proof'. Having a lot of examples of something being true is not the same as 'proof'. We don't give any proof yet, however. A worked example uses algebra to solving . Exercises include finding congruent triangles in this figure...
- The Pythagorean Theorem We define 'hypotenuse' and check the student can identify the hypotenuse, show how the formula works and how to rearrange it. We look at specific examples, the 30-60-90 and 45-90-45 triangles. Make sure they recognise them when in different orientations. We then show how to use Pythagoras' theorem more than once, with a steps in a spiral, and with the 'pyramid height' example. We remind that we have not yet proved that Pythagoras is true. Exercises.
- Proof: Pythagorean Theorem We give the pretty demonstration of Pythagoras to show why it's true followed by Euclid's proof.
- Exercise: A Puzzle Triangle Triangle puzzle (invented by Paul Curry). This is getting across the importance of rigour in a fun way. Students who do work out what is going on will have a deeper sense of why you need to be careful in a proof and justify every step. It is not enough that two things 'look' the same. Students who don't work out the explanation themselves will still have had practice at calculating area and using Pythagoras. Later when we've taught cosine we suggest they go back and calculate the angles, so they get a second chance. We've made this exercise easier by having talked about slopes in an earlier exercise, with the roadsigns, and even had the same close ratios in those slopes.
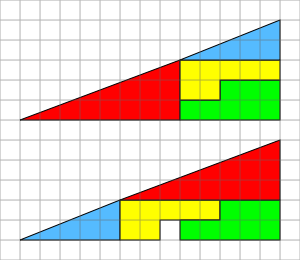 How diagrams can mislead
How diagrams can mislead - Proof: Angles sum to 180: We give the pretty demonstration of why this is so using image of triangle divided into four smaller triangles. Student asked to convince themselves it is still true when the triangle has an obtuse angle. Ensure that they know that a bigger triangle does not have 'bigger angles'. We follow with the trad proof.
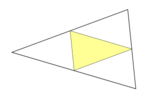 Angles sum to 180 - Demonstrate using this diagram
Angles sum to 180 - Demonstrate using this diagram - Exercise: Plotting (Cos t, Sin t) This is a really crucial page in the course, where we introduce students to and (in degrees). We ask the student to use their calculator and draw a table of some pairs for various values of , like 0,30,60,45,90,120,135... Where do they appear on a graph? Define and as x and y for points on a unit circle. Also pronunciation of sin/sine coz/cosine. Note that it is unusual to introduce cosine and sine this way, using the unit circle, but it is an easier route than starting from soh-cah-toa. There can be a sense of delight and discovery that the cosine and sine buttons on the calculator are for 'the unit circle'.
- Soh-Cah-Toa , using 30-60-90 triangle as an example. Then a 5-85-90 triangle! This relates it to the unit circle, and reminds that the cos of theta is the length of the adjacent side when the hypotenuse is one.
- Sine Squared plus Cosine Squared It's just Pythagoras in disguise. Explains the notation i.e. . Takes some time to explain that squaring a number between minus one and plus one makes it closer to zero.
- Radians We can extract some radian content from this page.
- The Unit Circle Circular chart and table of trig values (as radicals).
- Solving Triangles Given ASA Solving Triangles Given ASA
- Solving Triangles Given SAS Solving Triangles Given SAS
- The most Difficult Triangles to Solve What's the hardest triangle-solving problem we can think up?
- Worked Example: Area of a Roof Using real architect's plans.
- Worked Example: Oil Tanker Arriving in Port Showing the route it takes, and the distance travelled.
- Law of Sines Law of Sines
- Law of Cosines Law of Cosines
- Law of Tangents Law of Tangents
- Phase and Frequency
- Graphs of Sine, Cos and Tan
- Graph of Sine Squared Graph of
- Addition Formula for Sines Not just the formula, but also a detailed walk through of the proof. How would you come up with the necessary diagram?
- Addition Formula for Cosines
- Double Angle Formulas
- Waves in and out of Phase
- Beat Frequencies
- Cosecant, Secant, Cotangent Cosecant, Secant, Cotangent. As well as the formulae we also illustrate with triangles in which the adjacent is 1 or the opposite is 1. We're not great fans of these functions, or in particular the many identities that follow. At this level it is quite enough to mention the functions and then stick to 1/sin, 1/cos, 1/tan where we might otherwise use them.
- Graphs of Cosec, Sec and Cot
- Inverse Trigonometric Functions
- other stuff
- Remembering Trig Formulae Remembering formulae - NO Mnemonics here. This is all about checking that the formula makes sense. The page I've linked to has some formulae and we'd add discussion of how to remember them or derive them instantly.
Trigonometry Book 1: For Enthusiasts
Some of these are easier than others; help in making these accessible to kids much appreciated.
- Introduction
- Regular Polygons Sum of angles in a hexagon, and a one-million-a-gon, and the lengths of the sides. Dividing up a polygon at the centre, at one vertex or looking at the exterior angles, to show that there is more than one way to do the same thing.
- What Tessellations are Possible with Regular Polygons? Here we allow mixes like squares and octagons.
- The Distance from New York to Tokyo Uses latitude and longitude, and calculation of an arc length from the length of a chord.
- Lissajous Figures Lissajous figures
- The CORDIC Algorithm A nice geometrical presentation of how a computer can compute sin and cosine rapidly using a small table for the angles
- Pythagorean Triples Formula for generating Pythagorean triples
- Triangles on a Sphere Triangles on a sphere.
Trigonometry Book 2 Contents
Requires Book 1, greater fluency with algebra - because fewer steps are shown - and for some parts introductory familiarity with matrices and vectors is needed.
- Introduction
- Trigonometry/Geometric Definitions of Trig Functions
- Thales Theorem also explain same principle applies to any angle, not just 90 degress.
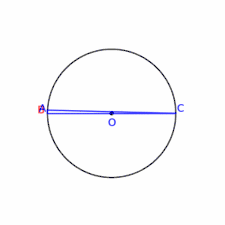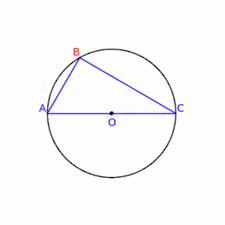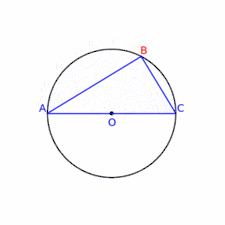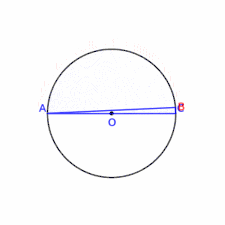 Animated illustration of Thales Theorem
Animated illustration of Thales Theorem - Ellipses Using sine and cosine to draw ellipses. Part of the presentation is an ellipse as a circle in perspective. We show that this is valid for both true perspective and orthographic projection (easier).
- Spirals Using and to draw spirals
- The Circumcircle Introduces the triple product and its interpretation.
- The Incircle
- The Excircles
- The Excentral Triangle
- The Pedal Triangle
- Distances Between Centres
- The Nine-point Circle
Trigonometry Book 2: For Enthusiasts
- The Delaunay Triangulation A good application of the Circumcircle.
- Doing without Sine
- Less-Used Trig Identities Treble (and higher) angle formulae, and some of the more beautiful but less useful trig identities, including these.
- Degrees Minutes and Seconds Degrees Minutes and Seconds (we don't need them in normal trig but should include them for completeness) I'm not really keen on this page, so hope someone else who is keen writes it.
Trigonometry Book 3 Contents
Rotations in 3D (including quaternions) will go into a wiki book on matrices, i.e not here. Probably Vectors and scalar and triple products belong there too.
- Introduction Intro - we're working in radians throughout. (Brief) review of complex numbers and differentiating polynomials.
- Derivative of cosine
- Derivative of Sine
- Power Series for e to the x Will start with and and derive the power series for . Remind that means 1x2x3x4=24. I'd like to refer to a new wikibook e-pi-phi-i which will go a bit deeper into (and and and ) and that will have lovely illustrations, especially for phi (spirals)
- Power Series for Cosine Then we will do the same trick for cos
- Power Series for Sine and then for sine
- Relating Power Series for Sine Cosine Exponential and then show that .
- A Square Wave in Sines Decomposing a square wave into sine waves
- The Gibbs Overshoot The Gibbs 'overshoot'.
- The Simple Harmonic Oscillator
Trigonometry Book 3: For Enthusiasts
- Trigonometry Done Rigorously Give a rigorous formulation of the Trigonometric functions, describe why calculus is usually involved. (The idea being that defining an angle involves the arc length for a circle, which is rigorously defined as an integral.)
- Chebyshev Polynomials Chebyshev polynomials are good approximations to the best (minimal max error) polynomials.
Teacher's Notes
- About This Book This page.
- Common Student Errors List the kinds of things to watch for.