Statics/Trusses

< Statics

Trusses are series of trianglular supports with no intraspan loads. Classified as two force members,the beams have applied forces only at the ends. The resultant forces at the ends must be equal in magnitude and opposite in direction, along the line of the joints of the member. These forces are called axial forces. The member is said to be in compression if T is negative (ie, the forces at each end are toward each other) or in tension if T is positive.

The main strategies for analyzing trusses are the method of joints and the method of sections.

How to die 101: Episode One - Engineering Trusses

Question leading to your death

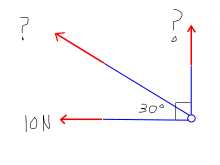
Blue lines are members of the truss, the blue circle is the joint which all members are attached to

The following picture shows a three member truss. Angles, and one force are given in the picture. Find the force in the vertical and diagonal member by using what you know about equilibrium.

I HATE TRUSSES!!!!! I want to die

Answer that immediately finishes your life

First, you want to dab and then hit that nae nae gurrllllll.

Then assign a coordinate system. In this problem a two dimensional X,Y plane can be used with the positive X axis being horizontal running from left to right(pointing to the right). The Y axis will be vertical and pointing upwards.

Since this is a statics problem, the joint cannot move. The only way the joint won't move is if the forces acting on it are in equilibrium (the net force must be equal to zero in all directions). An intelligent step now would be to sum X and Y forces at the joint.

Assign variable names so you don't get members confused with each other, let's call the vertical member's tension $T_{1}$ and the diagonal member's tension $T_{2}$ .

$\sum F_{x}=0=-10-T_{2}\cos(30)+T_{1}\cos(90)$

$\sum F_{y}=0=T_{1}+T_{2}\sin(30)$

The cosine of 90 degrees is zero so $T_{1}$ drops out. You could also drop out $T_{1}$ simply by noticing that $T_{1}$ does not have an X component.

$\sum F_{x}=0=-10-T_{2}\cos(30)$

$\ T_{2}=-11.547N$

The negative sign indicates that the force is in the opposite direction as in the picture. This means the member is in compression instead of tension. Now we can solve for $T_{1}$ .

$\sum F_{y}=0=T_{1}+T_{2}sin(30)$

$\sum F_{y}=0=T_{1}+-11.547sin(30)$

$\ T_{1}=5.774N$

This answer came out positive, meaning the direction indicated in the picture was correct and the member is in tension.

The problem has been solved, we know the force in every member of the truss and whether the member is in tension or compression. If you are having problems visualizing the problem see if the image below helps.

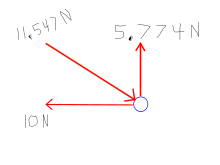
This picture displays every force acting on the joint in the correct orientation, you can see that the forces add to zero so the joint will not move.

FINALLY! Your done. Now stand up a scream to show your awesome talent.

Notes to further den your suffering

The moments about all axes must also be zero, but the moments are already known to be zero about the joint because all forces pass directly through the joint.

Quick links:Statics, Civil Engineering, Mechanical Engineering

This article is issued from Wikibooks. The text is licensed under Creative Commons - Attribution - Sharealike. Additional terms may apply for the media files.