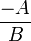Intermediate Algebra/Linear Equations
< Intermediate AlgebraLinear Equations
A linear equation is an equation that forms a line on a graph.
Slope-Intercept form
A linear equation in slope-intercept form is one in the form 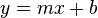 such that
such that 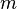 is the slope, and
is the slope, and 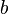 is the y-intercept. An example of such an equation is:
is the y-intercept. An example of such an equation is:
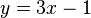
Finding y-intercept, given slope and a point
The y-intercept of an equation is the point at which the line produced touches the y-axis, or the point where  This can be very useful. If we know the slope, and a point which the line passes through, we can find the y-intercept. Consider:
This can be very useful. If we know the slope, and a point which the line passes through, we can find the y-intercept. Consider:
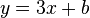 Which passes through
Which passes through 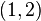
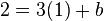 Substitute
Substitute 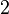 and
and  for
for 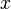 and
and 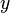 , respectively
, respectively
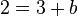 Simplify.
Simplify.

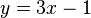 Put into slope-intercept form.
Put into slope-intercept form.
Finding slope, given y-intercept and a point
The slope of a line is defined as the amount of change in x and y between two points on the line.
If we know the y-intercept of the line, and a point on the line, we can easily find the slope. Consider:
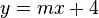 which passes through the point
which passes through the point 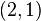
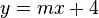
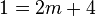 Replace
Replace 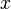 and
and 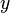 with
with  and
and 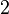 , respectively.
, respectively.
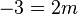 Simplify.
Simplify.
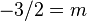
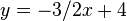 Put into slope-intercept form.
Put into slope-intercept form.
Standard form
The Standard form of a line is the form of a linear equation in the form of 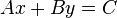 such that
such that 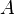 and
and 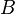 are integers, and
are integers, and 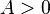 .
.
Converting from slope-intercept form to standard form
Slope-intercept equations can easily be changed to standard form. Consider the equation:
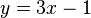
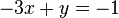 Subtract -3x from each side, satisfying
Subtract -3x from each side, satisfying 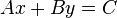
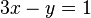 Multiply the entire equation by
Multiply the entire equation by 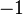 , satisfying
, satisfying 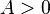
 and
and  are already integers, so we don't have to worry about changing them.
are already integers, so we don't have to worry about changing them.
Finding the slope of an equation in standard form
In the standard form of an equation, the slope is always equal to