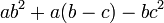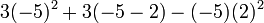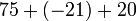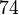Intermediate Algebra/Chapter1TestAnswers

Chapter 1 Test: Answers

1. 74; Substitute 3 for a, -5 for b, and c for 2, then use the order of operations to simplify:

 $ab^2+a(b-c)-bc^2$ 
 $3(-5)^2+3(-5-2)-(-5)(2)^2$ 

 $75+(-21)+20$ 

 $74$

2. 15.92 inches, to the nearest hundredth. Substitute the appropriate values into the formula (circumference = 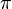$\pi$ * diameter) and solve the equation:

C = πd
50 ≈ 3.14d
d ≈ 15.92 inches

 a. N, Z, and Q:  $\sqrt{49} = 7$ , which falls under those three categories.
 b. Q: -3.84 is a terminating decimal.
 c. N, Z, and Q: 6 - (-3) = 9, a positive whole number, integer, and rational number.
 d. N, Z, and Q: ((2×5)/4) + (3/2) = (8/2), or 4.

 a. Distributive Property: The expression (x + 3) distributes 2 per term.
 b. Commutative Property of Addition: a + b = b + a
 c. Associative Property of Addition: a + (b + c) = (a + b) + c
 d. Inverse Property of Multiplication: The product of a number and its reciprocal is 1.

5. Use the Distributive Property and combine like terms for each expression. The results are worked out below:

 a. 3y(4 + 6x) - 2(y - 6) = 12y + 18xy - 2y + 12 = 10y + 18xy + 12
 b. 2(a - 5) + 3a = 2a - 10 + 3a = 5a - 10
 c. 5[c + 3(2c - 1)] = 5(c + 6c - 3) = 5(7c - 3) = 35c - 15

6. Let x = the number of books Melvin bought. If each stamp costs $0.39, each book contains one dozen (12) stamps, and Melvin pays an additional $1.40 sales tax, all combined for a total purchase of $24.80, then we can write the equation as follows:

 $0.39(12x) + 1.4 = 24.8$ 
Now solve using the Properties of Equality:

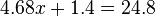
 $4.68x + 1.4 = 24.8$ 
 $4.68x = 23.4$ 
 $x = 5$ 

Therefore, Melvin bought 5 books of stamps.

|3y - 4| + 5 = 10.
|3y - 4| = 5 
3y - 4 = ±5
3y = 9 or 3y = -1
y = 3 or y = -(1/3)

a. 
2y - 4 > 3
2y > 7
y > 3.5

b.
-7x < 35 + 3x
-10x < 35
x > -3.5

c.
 $\frac{32}{-x - 2}\ > 4$ 
32 > 4(-x - 2)
32 > -4x - 8
40 > -4x
-10 > x

9. Let x = Necessary credit value. If we know that one of Jack's 7 courses has a credit value of 4, and Jack needs 40 credits in order to pass the year, then we can write the equation as follows:

 $6x + 4 = 40$ 
Now solve using the Properties of Equality:

 $6x = 36$ 
 $x = 6$

This article is issued from Wikibooks. The text is licensed under Creative Commons - Attribution - Sharealike. Additional terms may apply for the media files.