General Relativity/Raising and Lowering Indices

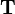Given a tensor $\mathbf{T}$ , the components 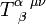$T^{\alpha \ \mu \nu}_{\ \beta}$ are given by 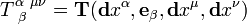$T^{\alpha \ \mu \nu}_{\ \beta}=\mathbf{T}(\mathbf{d}x^\alpha, \mathbf{e}_\beta, \mathbf{d}x^\mu, \mathbf{d}x^\nu)$ (just insert appropriate basis vectors and basis one-forms into the slots to get the components).

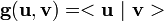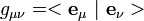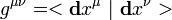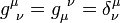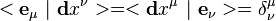So, given a metric tensor $\mathbf{g}(\mathbf{u}, \mathbf{v})=<\mathbf{u} \ |\ \mathbf{v}>$ , we get components $g_{\mu \nu}=<\mathbf{e}_\mu \ |\ \mathbf{e}_\nu>$ and $g^{\mu \nu}=<\mathbf{d}x^\mu \ |\ \mathbf{d}x^\nu>$ . Note that $g^\mu_{\ \nu}=g_\mu^{\ \nu}=\delta^\mu_\nu$ since $<\mathbf{e}_\mu \ |\ \mathbf{d}x^\nu>=<\mathbf{d}x^\mu \ |\ \mathbf{e}_\nu>=\delta^\mu_\nu$ .

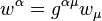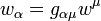Now, given a metric, we can convert from contravariant indices to covariant indices. The components of the metric tensor act as "raising and lowering operators" according to the rules $w^\alpha=g^{\alpha \mu}w_{\mu}$ and $w_\alpha=g_{\alpha \mu}w^\mu$ . Here are some examples:

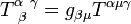
1. $T^{\alpha \ \gamma}_{\ \beta} = g_{\beta \mu}T^{\alpha \mu \gamma}$

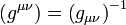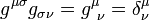Finally, here is a useful trick: thinking of the components of the metric as a matrix, it is true that $\left( g^{\mu \nu} \right) = \left( g_{\mu \nu} \right) ^{-1}$ since $g^{\mu \sigma}g_{\sigma \nu}=g^\mu_{\ \nu}=\delta^\mu_\nu$ .

This article is issued from Wikibooks. The text is licensed under Creative Commons - Attribution - Sharealike. Additional terms may apply for the media files.