General Chemistry/The Quantum Atom

The Quantum Numbers

These four numbers are used to describe the location of an electron in an atom.

Number	Symbol	Possible Values
Principal Quantum Number	$n \,$	$\displaystyle 1,2,3,4,\ldots$
Angular Momentum Quantum Number	$\ell \,$	$\displaystyle 0,1,2,3,\ldots ,(n-1)$
Magnetic Quantum Number	$m_{\text{l}}\,$	$\displaystyle -\ell ,\ldots ,-1,0,1,\ldots ,\ell \,$
Spin Quantum Number	$m_{\text{s}}\,$	$\displaystyle +1/2,-1/2$

Principal Quantum Number (n)

Determines the shell the electron is in. The shell is the main component that determines the energy of the electron (higher n corresponds to higher energy), as well as nuclear distance (higher n means further from the nucleus). The row that an element is placed on the periodic table tells how many shells there will be. Helium (n = 1), neon (n = 2), argon (n = 3), etc.

Angular Momentum Quantum Number (l)

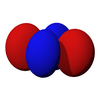
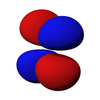
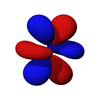
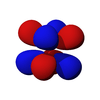
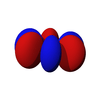
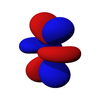
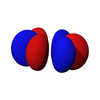
Also known as azimuthal quantum number. Determines the subshell the electron is in. Each subshell has a unique shape and a letter name. The s orbital is shaped like a sphere and occurs when l = 0. The p orbitals (there are three) are shaped like teardrops and occur when l = 1. The d orbitals (there are five) occur when l = 2. The f orbitals (there are seven) occur when l = 3. (By the way, when l = 4, the orbitals are "g orbitals", but they (and the l = 5 "h orbitals") can safely be ignored in general chemistry.)

This number also gives information as to what the angular node of an orbital is. A node is defined as a point on a standing wave where the wave has minimal amplitude. When applied to chemistry this is the point of zero-displacement and thus where no electrons are found. In turn angular node means the planar or conical surface in which no electrons are found or where there is no electron density.

Here are pictures of the orbitals. Keep in mind that they do not show the actual path of the electrons, due to the Heisenberg Uncertainty Principle. Instead, they show the area where the electron is most likely to occur (say, 90% of the probability). The two colors represent the two different spin numbers (the choice is arbitrary).

m₁	-3	-2	-1	0	1	2	3
S orbital →
P orbitals →
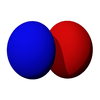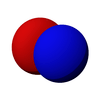D orbitals →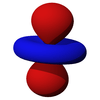
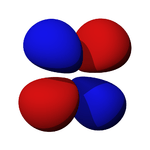F orbitals →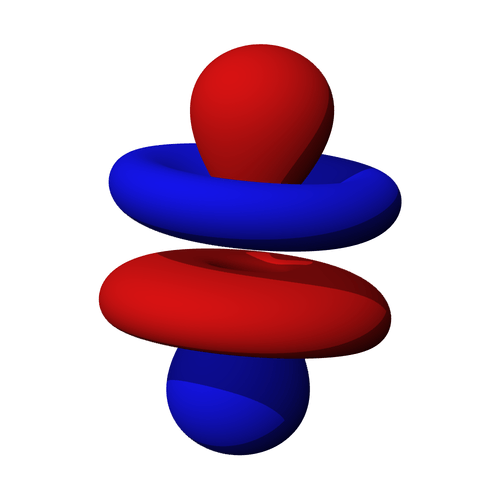

Magnetic Quantum Number (m_l)

Determines the orbital in which the electron lies. For example, there are three p orbitals in shell n = 2: the magnetic quantum number determines which one of these orbitals the electrons reside in. The different orbitals are oriented at different angles around the nucleus. See how each p orbital has the same general shape, but they point in different directions around the nucleus.

Spin Quantum Number (m_s)

Determines the spin on the electron. +½ corresponds to the up arrow in an electron configuration box. If there is only one electron in an orbital (one arrow in one box), then it is always considered +½. The second arrow, or down arrow, is considered -½.

Some Examples

Let's examine the quantum numbers of electrons from a magnesium atom, ¹²Mg. Remember that each list of numbers corresponds to (n, l, m_l, m_s).

Two s electrons:	(1, 0, 0, +½)	(1, 0, 0, -½)
Two s electrons:	(2, 0, 0, +½)	(2, 0, 0, -½)
Six p electrons:	(2, 1, -1, +½)	(2, 1, -1, -½)	(2, 1, 0, +½)	(2, 1, 0, -½)	(2, 1, 1, +½)	(2, 1, 1, -½)
Two s electrons:	(3, 0, 0, +½)	(3, 0, 0, -½)

The Periodic Table

Notice a pattern on the periodic table. Different areas, or blocks, have different types of electrons. The two columns on the left make the s-block. The six columns on the right make the p-block. The large area in the middle (transition metals) makes the d-block. The bottom portion makes the f-block (Lanthanides and Actinides). Each row introduces a new shell (aka energy level). Basically, the row tells you how many shells of electrons there will be, and the column tells you which subshells will occur (and which shells they occur in). The value of m_l can be determined by some of the rules we will learn in the next chapter. The value of m_s doesn't really matter as long as there are no repeating values in the same orbital.

This article is issued from Wikibooks. The text is licensed under Creative Commons - Attribution - Sharealike. Additional terms may apply for the media files.