Fundamentals of Transportation/Trip Generation/Solution

< Fundamentals of Transportation < Trip Generation

Problem:

Planners have estimated the following models for the AM Peak Hour

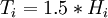
$T_i = 1.5*H_i \,\!$

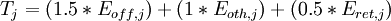
$T_j = (1.5*E_{off,j}) + (1*E_{oth,j}) + (0.5*E_{ret,j}) \,\!$

Where:

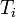$T_i\,\!$ = Person Trips Originating in Zone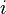 $i\,\!$

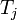$T_j\,\!$ = Person Trips Destined for Zone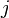 $j\,\!$

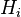$H_i\,\!$ = Number of Households in Zone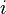 $i\,\!$

You are also given the following data

Data
Variable	Dakotopolis	New Fargo
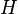$H$	10000	15000
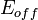$E_{off}$	8000	10000
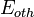$E_{oth}$	3000	5000
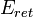$E_{ret}$	2000	1500

A. What are the number of person trips originating in and destined for each city?

B. Normalize the number of person trips so that the number of person trip origins = the number of person trip destinations. Assume the model for person trip origins is more accurate.

Solution:

A. What are the number of person trips originating in and destined for each city?

Solution to Trip Generation Problem Part A
	Households (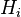 $H_i$ )	Office Employees (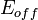 $E_{off}$ )	Other Employees (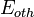 $E_{oth}$ )	Retail Employees (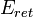 $E_{ret}$ )	Origins 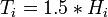$T_i = 1.5*H_i$	Destinations 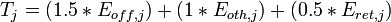$T_j = (1.5E_{off,j}) + (1E_{oth,j}) + (0.5*E_{ret,j})$
Dakotopolis	10000	8000	3000	2000	15000	16000
New Fargo	15000	10000	5000	1500	22500	20750
Total	25000	18000	8000	3000	37500	36750

B. Normalize the number of person trips so that the number of person trip origins = the number of person trip destinations. Assume the model for person trip origins is more accurate.

Use: 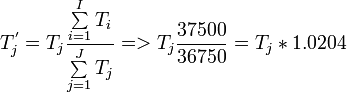$\quad T_j^' = T_j \frac{{\sum\limits_{i = 1}^I {T_i } }}{{\sum\limits_{j = 1}^J {T_j } }} = > T_j \frac{{37500}}{{36750}} = T_j *1.0204 \,\!$

Solution to Trip Generation Problem Part B
	Origins (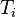 $T_i$ )	Destinations (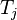 $T_j$ )	Adjustment Factor	Normalized Destinations (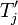 $T_j'$ )	Rounded
Dakotopolis	15000	16000	1.0204	16326.53	16327
New Fargo	22500	20750	1.0204	21173.47	21173
Total	37500	36750	1.0204	37500	37500

This article is issued from Wikibooks. The text is licensed under Creative Commons - Attribution - Sharealike. Additional terms may apply for the media files.