Fundamentals of Transportation/Shockwaves/Solution

< Fundamentals of Transportation < Shockwaves

Problem:

Flow on a road is 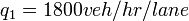$q_1=1800 veh/hr/lane$ , and the density of 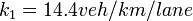$k_1=14.4 veh/km/lane$ . To reduce speeding on a section of highway, a police cruiser decides to implement a rolling roadblock, and to travel in the left lane at the speed limit ( 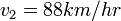$v_2=88 km/hr$ ) for 10 km. No one dares pass. After the police cruiser joins, the platoon density increases to 20 veh/km/lane and flow drops. How many vehicles (per lane) will be in the platoon when the police car leaves the highway?

How long will it take for the queue to dissipate?

Solution:

Step 0

Solve for Unknowns:

Original speed

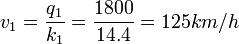
$v_1=\frac{q_1}{k_1}=\frac{1800}{14.4} = 125 km/h \,\!$

Flow after police cruiser joins

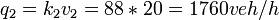
$q_2= k_2 v_2 = 88*20 = 1760 veh/h \,\!$

Step 1

Calculate the wave velocity:

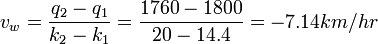
$v_w = \frac{{q_2 - q_1 }}{{k_2 - k_1 }} = \frac{{1760 - 1800}}{{20 - 14.4}} = - 7.14km/hr \,\!$

Step 2

Determine the growth rate of the platoon (relative speed)

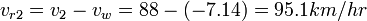
$v_{r2} = v_2 - v_w = 88 - ( - 7.14) = 95.1km/hr \,\!$

Step 3

Determine the time spent by the police cruiser on the highway

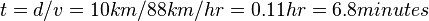
$t = d/v = 10 km / 88 km/hr = 0.11 hr = 6.8 minutes \,\!$

Step 4

Calculate the Length of platoon (not a standing queue)

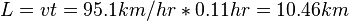
$L = v t = 95.1 km/hr * 0.11 hr = 10.46 km \,\!$

Step 5

What is the rate at which the queue grows, in units of vehicles per hour?

$\Delta{q} = q_1 - k_1 v_w = q_2 - k_2 v_w = 1800 - (14.4*-7.14) = 1760- (20*-7.14) = 1902 veh/hr \,\!$

Step 6

The number of vehicles in platoon

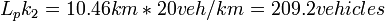
$L_p k_2 = 10.46 km * 20 veh/km = 209.2 vehicles \,\!$

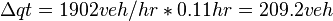
$\Delta{q} t = 1902 veh/hr*0.11hr=209.2veh \,\!$

How long will it take for the queue to dissipate?

(a) Where does the shockwave go after the police cruiser leaves? Just reverse everything?

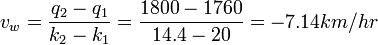
${{v}_{w}}=\frac{{{q}_{2}}-{{q}_{1}}}{{{k}_{2}}-{{k}_{1}}}=\frac{1800-1760}{14.4-20}=-7.14km/hr$

Second wave never catches first.

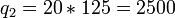
(b) Return speed to 125, keep density @ 20? $q_2 = 20*125=2500 \,\!$

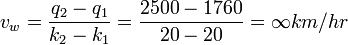
${{v}_{w}}=\frac{{{q}_{2}}-{{q}_{1}}}{{{k}_{2}}-{{k}_{1}}}=\frac{2500-1760}{20-20}=\infty km/hr$

Second wave instantaneously catches first

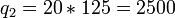
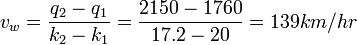
${{v}_{w}}=\frac{{{q}_{2}}-{{q}_{1}}}{{{k}_{2}}-{{k}_{1}}}=\frac{2150-1760}{17.2-20}=139km/hr$

Second wave quickly catches first

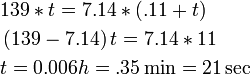
$\begin{align} & 139*t=7.14*\left( .11+t \right) \\ & \left( 139-7.14 \right)t=7.14*11 \\ & t=0.006h=.35\min =21\sec \\ \end{align}$

(d) Drop upstream q,k to slow down formation curve.

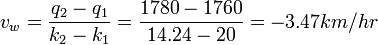
${{v}_{w}}=\frac{{{q}_{2}}-{{q}_{1}}}{{{k}_{2}}-{{k}_{1}}}=\frac{1780-1760}{14.24-20}=-3.47km/hr$

Second wave eventually catches first

(e) If 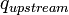$q_{upstream}$ falls below downstream capacity, wave dissipates

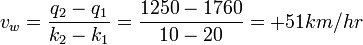
${{v}_{w}}=\frac{{{q}_{2}}-{{q}_{1}}}{{{k}_{2}}-{{k}_{1}}}=\frac{1250-1760}{10-20}=+51km/hr$

Forward moving wave.

This article is issued from Wikibooks. The text is licensed under Creative Commons - Attribution - Sharealike. Additional terms may apply for the media files.