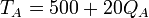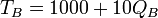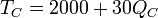Fundamentals of Transportation/Route Choice/Homework

< Fundamentals of Transportation < Route Choice

Homework

1. If trip distribution depends on travel times, and travel times depend on the trip table (resulting from trip distribution) that is assigned to the road network, how do we solve this problem (conceptually)?

2. Do drivers behave in a system optimal or a user optimal way? How can you get them to move from one to the other.

3. Identify a mechanism that can ensure the system optimal outcome is achieved in route assignment, rather than the user equilibrium. Why would we want such an outcome? What are the drawbacks to the mechanism you identified?

4. Assume the flow from Dakotopolis to New Fargo, is 5300 vehicles per hour. The flow is divided between two parallel facilities, a freeway and an arterial. Flow on the freeway is denoted 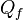$Q_f$ , and flow on the two-lane arterial is denoted 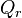$Q_r$ . The travel time on the freeway ( 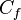$C_f$ ) is given by:

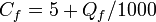
$C_f = 5 + Q_f/1000$

The travel time on the arterial (Cr) is given by

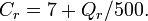
$C_r = 7 + Q_r/500.$

(a) Apply Wardrop's User Equilibrium Principle, and determine the flow and travel time on both routes from Dakotopolis to New Fargo.

(b) Solve for the System Optimal Solution and determine the flow and travel time on both routes.

5. Given a flow of 10,000 vehicles from origin to destination traveling on three parallel routes. Flow on each route A, B, or C is designated with 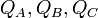$Q_A, Q_B, Q_C$ in the Time Function Respectively. Apply Wardrop's Network Equilibrium Principle (Users Equalize Travel Times on all used routes), and determine the flow on each route.

$T_A = 500 + 20Q_A$

$T_B = 1000 + 10Q_B$

$T_C = 2000 + 30Q_C$

This article is issued from Wikibooks. The text is licensed under Creative Commons - Attribution - Sharealike. Additional terms may apply for the media files.