Fundamentals of Transportation/Queueing/Solution3

< Fundamentals of Transportation < Queueing

Problem:

In this problem we apply the properties of capacitated queues to an expressway ramp. Note the following:

Ramp will hold 15 vehicles
Vehicles can enter expressway at 1 vehicle every 6 seconds
Vehicles arrive at ramp at 1 vehicle every 8 seconds

Determine:

(A) Probability of 5 cars,

(B) Percent of Time Ramp is Full,

Solution:

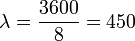
$\lambda = \frac{{3600}}{8} = 450 \,\!$

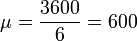
$\mu = \frac{{3600}}{6} = 600 \,\!$

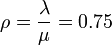
$\rho = \frac{\lambda }{\mu } = 0.75 \,\!$

Part A

Probability of 5 cars,

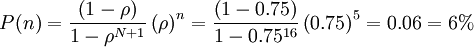
$P(n) = \frac{{\left( {1 - \rho } \right)}}{{1 - \rho ^{N + 1} }}\left( \rho \right)^n = \frac{{\left( {1 - 0.75} \right)}}{{1 - 0.75^{16} }}\left( {0.75} \right)^5 = 0.06 = 6\% \,\!$

Part B

Percent of time ramp is full (i.e. 15 cars),

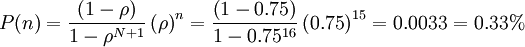
$P(n) = \frac{{\left( {1 - \rho } \right)}}{{1 - \rho ^{N + 1} }}\left( \rho \right)^n = \frac{{\left( {1 - 0.75} \right)}}{{1 - 0.75^{16} }}\left( {0.75} \right)^{15} = 0.0033 = 0.33\% \,\!$

Part C

Expected number of vehicles on ramp in peak hour.

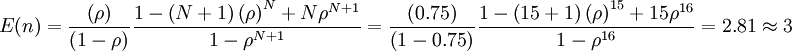
$E(n) = \frac{{\left( \rho \right)}}{{\left( {1 - \rho } \right)^{} }}\frac{{1 - \left( {N + 1} \right)\left( \rho \right)^N + N\rho ^{N + 1} }}{{1 - \rho ^{N + 1} }} = \frac{{\left( {0.75} \right)}}{{\left( {1 - 0.75} \right)^{} }}\frac{{1 - \left( {15 + 1} \right)\left( \rho \right)^{15} + 15\rho ^{16} }}{{1 - \rho ^{16} }} = 2.81 \approx 3 \,\!$

Conclusion, ramp is large enough to hold most queues, though 12 seconds an hour, there will be some ramp spillover. It is a policy question as to whether that is acceptable.

This article is issued from Wikibooks. The text is licensed under Creative Commons - Attribution - Sharealike. Additional terms may apply for the media files.