Fundamentals of Transportation/Evaluation/Solution

< Fundamentals of Transportation < Evaluation

Problem:

A new transportation project is proposed to the city. This project is a form of "guide wire", where cars can hook to these moving, below-ground wires and be transported for free around town. This project is proven to reduce gas consumption by $5 million in its completion year. The city's preferred contractor says that it will take 10 years to build the thing and cost $470,000 a year, which is "perfect" because benefit would exceed costs by more than $300,000. Knowing that inflation is 3 percent, as an expert evaluator, is this a wise decision?

Solution:

Convert everything to Present Value and see just how great a deal this is.

For the present value of the $5,000,000 benefit (gas reduction):

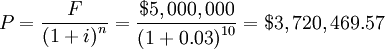
$P = \frac{F}{{\left( {1 + i} \right)^n }} = \frac{$5,000,000}{{\left( {1 + 0.03} \right)^{10} }} = $3,720,469.57 \,\!$

The benefit in present-day value is $3,720,469.57.

For the money (cost) being sent to the contractor, a payment of $470,000 per year, the present value would be:

$P = A\left[ {\frac{{\left( {1 + i} \right)^n - 1}}{{i\left( {1 + i} \right)^n }}} \right] = $470,000\left[ {\frac{{\left( {1 + 0.03} \right)^{10} - 1}}{{0.03\left( {1 + 0.03} \right)^{10} }}} \right] = $4,009,195.33 \,\!$

The cost in present-day value is $4,009,195.33. Therefore, this detail, while shiny in appearance at first, is NOT a wise decision, since costs exceed benefits.

This article is issued from Wikibooks. The text is licensed under Creative Commons - Attribution - Sharealike. Additional terms may apply for the media files.