Fundamentals of Transportation/Earthwork/Solution

Problem:

Given the end areas below, calculate the volumes of cut (in cubic meters) and fill between stations 0+00 and 1+50. Determine the true amount of excess cut or fill to be removed.

• 0+00: Fill = 60
• 0+50: Fill = 50
• 0+75: Cut = 0, Fill = 25
• 1+00: Cut = 10, Fill = 5
• 1+15: Cut = 15, Fill = 0
• 1+50: Cut = 30

Solution:

Two different methods need to be used here to compute earthwork volumes along the five strips. The average end area method can be used for non-zero sections. The pyramid method needs to be used for areas with zero ends.

For 0+00 to 0+50, use average end area:

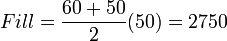
For 0+50 to 0+75, use average end area:

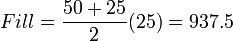
For 0+75 to 1+00, use the average end area method for the fill section and the pyramid method for the cut section:

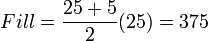
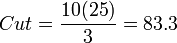
For 1+00 to 1+15, use the pyramid method for the fill section and the average end area method for the cut section:

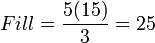
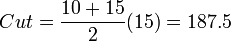
For 1+15 to 1+50, use the average end area method:

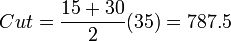
The sums of both cut and fill can be found:

• Fill = 4087.5 cubic-meters
• Cut = 1058.3 cubic-meters

Thus, 3029.2 cubic-meters of dirt are needed to meet the earthwork requirement for this project.