Fractals/book
< FractalsBook here means :
- unnamed c program[1] by Claude Heiland-Allen[2]
- one gui program
- set of the console programs
- book in pdf format about : " how to write a book about the Mandelbrot set ". Here is unofficial version of pdf file ( 68.3 MB )
Features
- zoom with automatic dynamic precision
- free c source code
- uses Newton method to compute :
Plane description
Radius is defined as "the difference in imaginary coordinate between the center and the top of the axis-aligned view rectangle". Radius has no initial value.
// / code/bin/mandelbrot_render.c
int retval = 1;
int width = 1280;
int height = 720;
int maximum_iterations = 4096;
long double escape_radius = 512;
mpfr_t radius;
mpfr_init2(radius, 53);
Algorithms
Images with full src code :
 level sets of escape time ( = exterior ) of Mandelbrot set
level sets of escape time ( = exterior ) of Mandelbrot set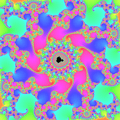 continous escape time
continous escape time atom domains algorithm
atom domains algorithm
- exterior :
- Smooth colouring with continuous escape time
- grid based on integer escape time and binary decomposition
- Atom domains
- external rays : the Newton Method[3]
- boundary : distance estimation ( DEM/M)
- interior :
All algorithms are described in the book : "How To Write A Book About The Mandelbrot Set" by Claude Heiland-Allen[6]
nucleus or center
See function mandelbrot_nucleus function from book/code/gui/main.c[7]. It uses Newton method.
Compare with the muatom() function in mightymandel/src/atom.c[8]
instal
dependencies
- OpenGl
- GL/glew.h ( libglew-dev )
- Gtk ( GUI
sudo apt install mesa-utils sudo apt install libglew-dev sudo apt install freeglut3-dev sudo apt-get install libgtk-3-dev
clone
First clone the official git repository :
git clone http://code.mathr.co.uk/book.git
or you can use unofficial repository :
git clone https://gitlab.com/adammajewski/my-book
make
go to the program directory and install :
cd book # or my_book cd code make -C lib make -C bin make cd gui make cd ..
make pdf
First
sudo apt-get install texlive-latex-recommended texlive-latex-extra texlive-fonts-recommended texlive-fonts-extra sudo apt-get install texlive-science
then to make book in pdf format :
- go to book/book directory
- build
cd book make
or use unofficial version with full c code[9]
run
There are 2 types of the last versions of book program:
- console
- gui
To run gui program:
# cd gui ./mandelbrot_gui
To run console program use bash scripts, see examples :
./examples/standardview.sh
Examples
- for the console program are bash sh files
- for gui program are text files
for console program
#!/bin/bash
# Radius is defined as "the difference in imaginary coordinate between the center and the top of the axis-aligned view rectangle".
# https://en.wikibooks.org/wiki/Fractals/Computer_graphic_techniques/2D/plane
#
# in examples directory : chmod +x standardview.sh
# then go to the parent directory : cd ..
# run run these example from the parent directory :
# ./examples/standardview.sh
#
# result :
# ./render using double
# rgba 1.000000 1.000000 1.000000 1.000000
# Image standard.png is saved
# info text file .txt is saved
view="-0.75 0 1.5" # "standard" view of parameter plane : center_re, center_im, radius
#
filename="standard"
pngfilename=$filename".png"
# Heredoc
./render $view && ./colour > out.ppm && ./annotate out.ppm $pngfilename <<EOF
rgba 1 1 1 1
EOF
echo "Image " $pngfilename " is saved"
# info text file
echo "view = re(center) im(center) radius = " $view > $filename".txt"
echo "info text file " $filname".txt is saved"
Extended use :
# Radius is defined as "the difference in imaginary coordinate between the center and the top of the axis-aligned view rectangle".
# https://en.wikibooks.org/wiki/Fractals/Computer_graphic_techniques/2D/plane
#
# in examples directory : chmod +x standardview2.sh
# then go to the parent directory : cd ..
# run run these example from the parent directory :
# ./examples/ian.sh
#
# result :
# ./render using double
# rgba 1.000000 1.000000 1.000000 1.000000
# Image standard.png is saved
# info text file .txt is saved
# from file : / code/bin/mandelbrot_render.c
# int width = 1280;
# int height = 720;
# standard view of parameter plane : center_re, center_im, radius
# plane description : view = center and radius
center_re="-1.86057396001587505"
center_im="-0.00000093437424500"
center="$center_re $center_im"
radius="3.5884751097983668e-09" #$(echo 1.0/$zoom | bc -l) # real radius
view="$center $radius"
# inbits are proportional to zoom
# inbits=$((54+$((4*$zoom))))
# image file names
filename="ian" # filename stem : stem="standard"
ppmfilename=$filename".ppm"
pngfilename=$filename".png"
# escape radius
er="512"
# image size in pixels
w="800"
h="600"
# maximum iterations
n="1500"
# interior rendering
i="1"
# Heredoc
# ./render $view && ./colour "$stem" > "$stem.ppm"
#./render $view "$er" "$filename" "$w" "$h" "$n" "$i" && ./colour "$filename" > "$ppmfilename" && ./annotate "$ppmfilename" $pngfilename <<EOF
#rgba 1 1 1 1
#EOF
echo "Image " $pngfilename " is saved"
# info text file
textfilename=$filename".txt"
ratio=$(echo $w/$h | bc -l)
# http://stackoverflow.com/questions/12882611/how-to-get-bc-to-handle-numbers-in-scientific-aka-exponential-notation
# bash do not use floating point
rim=`echo ${radius} | sed -e 's/[eE]+*/\\*10\\^/'` # conver e to 10
rre=$(echo $ratio*$rim | bc -l) # real radius
cu=$(echo $center_im+$rim | bc -l)
cd=$(echo $center_im-$rim | bc -l)
cl=$(echo $center_re+$rre | bc -l)
cr=$(echo $center_re-$rre | bc -l)
rim="("$rim")" # add () because precedence of operators
zoom=$(echo 1/$rim | bc -l) # zoom = 1/radius
z=$(echo "$zoom" | sed 's/e/*10^/g;s/ /*/' | bc)
echo "part of parameter complex plane " > $textfilename
echo "center of image c = " $center >> $textfilename
echo "radius = (image height/2) = " $rim >> $textfilename
echo "radius = (image height/2) = " $radius >> $textfilename
echo "up corner = center_im+radius = cu =" $cu >> $textfilename
echo "down corner = center_im-radius = cd =" $cd >> $textfilename
echo "left corner = center_re+ratio*radius = cl =" $cl >> $textfilename
echo "right corner = center_im-ratio*radius = cr =" $cr >> $textfilename
echo "image ratio = width/height =" $ratio >> $textfilename
echo "zoom level = fractint mag = "$zoom >>$textfilename
echo "ratio = image width/height = " $ratio >> $textfilename
echo "info text file " $textfilename "is saved"
helper programs
period
usage :
./mandelbrot_box_period cx cy radius maxperiod
example :
./mandelbrot_box_period 3.06095924635699068e-01 2.37427672737983361e-02 2.0000000000000001e-10 1000
next example :
./mandelbrot_box_period -0.220857943592514 -0.650752006989254 0.0000020 2000 596
Compute nucleus
usage:
./mandelbrot_nucleu bits cx cy period maxiters
example :
./mandelbrot_nucleus 100 3.06095924635699068e-01 2.37427672737983361e-02 68 1000
angles
mandelbrot_external_angles
Compute angles of rays landing on the root point,[10] usage:
./mandelbrot_external_angles bits cx cy period
example :
./mandelbrot_external_angles 100 3.06095924635699068e-01 2.37427672737983361e-02 68
New :
./mandelbrot_external_angles 53 -3.9089629378291085e-01 5.8676031775674931e-01 89
result :
.(01001010010010100101001001010010010100101001001010010100100101001001010010100100101001001) .(01001010010010100101001001010010010100101001001010010100100101001001010010100100101001010)
Another :
./mandelbrot_external_angles 100 -1.115234269232491 0.252761823831669 24 .(010110010110010110011001) .(010110010110011001010110)
mandelbrot_describe_external_angle

G Pastor gave an example of external rays for which the resolution of the IEEE 754 is not sufficient [11]:
( period 3, lands on root point of period 3 component c3 = -0.125000000000000 +0.649519052838329i )
One can analyze these angles using program by Claude Heiland-Allen :
./bin/mandelbrot_describe_external_angle ".(001)"
binary: .(001)
decimal: 1/7
preperiod: 0
period: 3
./bin/mandelbrot_describe_external_angle
".(001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001010)"
binary:
.(001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001010)
decimal:
33877456965431938318210482471113262183356704085033125021829876006886584214655562/237142198758023568227473377297792835283496928595231875152809132048206089502588927
preperiod: 0
period: 267
./bin/mandelbrot_describe_external_angle
".(001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001010001)"
binary:
.(001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001001010001)
decimal:
33877456965431938318210482471113262183356704085033125021829876006886584214655569/237142198758023568227473377297792835283496928595231875152809132048206089502588927
preperiod: 0
period: 267
./bin/mandelbrot_describe_external_angle
".(0010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010001)"
binary:
.(0010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010001)
decimal:
67754913930863876636420964942226524366713408170066250043659752013773168429311121/474284397516047136454946754595585670566993857190463750305618264096412179005177855
preperiod: 0
period: 268
./bin/mandelbrot_describe_external_angle
".(0010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010)"
binary:
.(0010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010010)
decimal:
67754913930863876636420964942226524366713408170066250043659752013773168429311122/474284397516047136454946754595585670566993857190463750305618264096412179005177855
preperiod: 0
period: 268
Landing points of above rays are roots with angled internal addresses ( description by Claude Heiland-Allen) :
- the upper one will be 1 -> 1/3 -> 3 -> 1/(period/3) -> period because it's the nearest bulb to the lower root cusp of 1/3 bulb and child bulbs of 1/3 bulb have periods 3 * denominator(internal angle) ie, 1 -> 1/3 -> 3 -> 1/89 -> 267
- the lower one will be 1 -> floor(period/3)/period -> period because it's the nearest bulb below the 1/3 cusp ie, 1 -> 89/268 -> 268
- the middle ray .(001) lands at the root of 1 -> 1/3 -> 3, from the cusp on the lower side (which is on the right in a standard unrotated view)
for gui program
size 2000 2000 view 53 3.0609592463186358e-01 2.3742767274521188e-02 7.6870929325598169e-11 text 57 3.060959246472445584e-01 2.374276727158354376e-02 272 text 56 3.06095924633099439e-01 2.3742767284688944e-02 204 text 56 3.06095924629285095e-01 2.37427672645622342e-02 204 text 54 3.06095924643046857e-01 2.374276727237906e-02 136 text 54 3.06095924629536442e-01 2.37427672749394494e-02 68 ray_in 2200 .(00000000000100000000000000110000000000000000000000000100000000000001) ray_in 2200 .(00000000000100000000000000101111111111111100000000000010000000000010)
To understand loading parameter files see deserialize function :
static int deserialize(const char *filename) {
FILE *in = fopen(filename, "rb");
if (in) {
while (G.anno) {
GtkTreeIter iter;
gpointer *thing;
gtk_tree_model_get_iter_first(GTK_TREE_MODEL(G.annostore), &iter);
if (gtk_tree_model_iter_next(GTK_TREE_MODEL(G.annostore), &iter)) {
gtk_tree_model_get(GTK_TREE_MODEL(G.annostore), &iter, 1, &thing, -1);
if (thing) {
delete_annotation((struct annotation *) thing);
gtk_tree_store_remove(G.annostore, &iter);
}
}
}
char *line = 0;
size_t linelen = 0;
ssize_t readlen = 0;
while (-1 != (readlen = getline(&line, &linelen, in))) {
if (readlen && line[readlen-1] == '\n') {
line[readlen - 1] = 0;
}
if (0 == strncmp(line, "size ", 5)) {
int w = 0, h = 0;
sscanf(line + 5, "%d %d\n", &w, &h);
// resize_image(w, h); // FIXME TODO make this work...
} else if (0 == strncmp(line, "view ", 5)) {
int p = 53;
int set_result;
char *xs = malloc(readlen);
char *ys = malloc(readlen);
char *rs = malloc(readlen);
sscanf(line + 5, "%d %s %s %s", &p, xs, ys, rs);
struct view *v = view_new();
mpfr_set_prec(v->cx, p);
mpfr_set_prec(v->cy, p);
set_result = mpfr_set_str(v->cx, xs, 0, GMP_RNDN);
set_result = set_result + mpfr_set_str(v->cy, ys, 0, GMP_RNDN);
set_result = set_result + mpfr_set_str(v->radius, rs, 0, GMP_RNDN);
free(xs);
free(ys);
free(rs);
if (set_result < 0) { printf("view line not valid, check chars\n"); return 1; }
start_render(v);
} else if (0 == strncmp(line, "ray_out ", 8)) {
int p = 53;
int set_result;
char *xs = malloc(readlen);
char *ys = malloc(readlen);
sscanf(line + 8, "%d %s %s", &p, xs, ys);
mpfr_t cx, cy;
mpfr_init2(cx, p);
mpfr_init2(cy, p);
set_result = mpfr_set_str(cx, xs, 0, GMP_RNDN);
set_result = set_result + mpfr_set_str(cy, ys, 0, GMP_RNDN);
free(xs);
free(ys);
double x = 0, y = 0;
param_to_screen(&x, &y, cx, cy);
mpfr_clear(cx);
mpfr_clear(cy);
if (set_result < 0) { printf("ray_out line not valid, check chars\n"); return 1; }
start_ray_out(x, y);
} else if (0 == strncmp(line, "ray_in ", 7)) {
int depth = 1000;
char *as = malloc(readlen);
sscanf(line + 7, "%d %s", &depth, as);
struct mandelbrot_binary_angle *angle = mandelbrot_binary_angle_from_string(as);
struct mandelbrot_binary_angle *angle2 = mandelbrot_binary_angle_canonicalize(angle);
mandelbrot_binary_angle_delete(angle);
start_ray_in(angle2, depth);
free(as);
} else if (0 == strncmp(line, "text ", 5)) {
int p = 53;
int set_result;
char *xs = malloc(readlen);
char *ys = malloc(readlen);
char *ss = malloc(readlen);
sscanf(line + 5, "%d %s %s %s", &p, xs, ys, ss);
struct annotation *anno = calloc(1, sizeof(struct annotation));
anno->tag = annotation_text;
anno->label = ss;
mpfr_init2(anno->u.text.x, p);
mpfr_init2(anno->u.text.y, p);
set_result = mpfr_set_str(anno->u.text.x, xs, 0, GMP_RNDN);
set_result = set_result + mpfr_set_str(anno->u.text.y, ys, 0, GMP_RNDN);
free(xs);
free(ys);
if (set_result < 0) {
free(ss);
mpfr_clear(anno->u.text.x);
mpfr_clear(anno->u.text.y);
printf("text line not valid, check chars \n");
return 1;
}
add_annotation(anno);
}
}
free(line);
fclose(in);
return 0;
} else {
return 1;
}
}
Images
Dictionary
bulb
- component
- Mu-Atom
Bond
"Bond is a " point where two mu-atoms meet. The parent "binds to" the child through the bond, and the two are said to be adjacent. This use of the word 'bond' was introduced by Benoit Mandelbrot in his description of the Mandelbrot set in The Fractal Geometry of Nature." From the Mandelbrot Set Glossary and Encyclopedia, by Robert Munafo, (c) 1987-2015.
In the book program result of computing bond is the internal angle ( = combinatorial rotation number ) of the parent components
dwell
"Dwell is a colloquial name for the Representation Function called Escape-Iterations." From the Mandelbrot Set Glossary and Encyclopedia, by Robert Munafo, (c) 1987-2015.
"The dwell bands are the regions of solid color that appear in a standard view of the Mandelbrot Set; they are also called level sets. All points in a dwell band have the same dwell. They are separated from one another by the "contour lines" or lemniscates."
hub
- branch type Misiurewicz point
nucleus
Nucleus of a Mu-Atom = center of hyperbolic component of Mandelbrot set
"The unique point within any mu-atom which has the property of belonging to its own limit cycle. This point is called the superstable point. This use of the word 'nucleus' was introduced by Benoit Mandelbrot in his description of the Mandelbrot set in The Fractal Geometry of Nature. If you set the polynomial formula for a lemniscate ZN equal to zero and solve for C (to get the roots of the polynomial), the roots are the nuclei of the mu-atoms of period N, plus any mu-atoms of periods that divide evenly into N. This procedure has been used numerically by Jay Hill to find all mu-atoms for periods up to about 16." From the Mandelbrot Set Glossary and Encyclopedia, by Robert Munafo, (c) 1987-2015.
Finding nucleus
A nucleus n of period p is a point of parameter plane
which satisfies :
where :
Claude is using Newton's method in one variable to compute c=n
Partials
the partials, namely the values of p for which |fpc(0)| reach a new minimum. It is related with the interior distance[12]
"Partials" is my term for the set of n with 0 < n such that |z_n| < |z_m| for all 0 < m < n, where z_{n+1} = z_n^2 + c, z_0 = 0. This is related to "atom period domain" colouring: http://mrob.com/pub/muency/atomdomain.html
A few examples:
-9.82053364278e-2 + 0.87751161636 i is the center of a bulb with period 30 and approximate size 8.7e-4 its partials are 1 3 6 12 30
-1.40107054826248 + 1.68078322683058e-2 i is the center of a cardioid with period 25 and approximate size 2.9e-7 its partials are 1 2 4 8 25
-6.30751837180080329933379814864882594423413629277790243935409e-02 + 9.97813152226579778761450011018468925066022924931316287706002e-01 i is the center of a cardioid with period 660 and approximate size 1e-51 its partials are 1 3 4 5 12 34 133 660
-1.949583466095265215576424927006606703613013182307914337344552997126238598475224082315026579e+00 + -7.76868505224924928703440073040924718407938044210292978384443422263629366944037056031909997e-06 i is the center of a cardioid with period 1820 and approximate size 3.5e-83 its partials are 1 2 3 4 9 25 83 359 1820
final ...
int final_n; // last iteration
complex float final_z; //
if (cabs2(z) > escape_radius2) {
final_n = n;
final_z = z; }
spoke
spoke = branch of the tree , arm of dendritic structure
How to contribute ?
git add book.pdf book.tex code/*.c git commit -m "description" git format-patch origin/master
and send patch to Claude by e-mail.
gcc render.c -E -o 26render.c remove some code manually add include
References
- ↑ c program by Claude Heiland-Allen
- ↑ Claude Heiland-Allen - blog
- ↑ An algorithm to draw external rays of the Mandelbrot set by Tomoki KAWAHIRA
- ↑ Interior coordinates in the Mandelbrot set
- ↑ Modified Atom Domains
- ↑ Mandelbrot Notebook
- ↑ book/code/gui/main.c
- ↑ mightymandel/src/atom.c code
- ↑ unofficial version ( with full c code ) of book "How to write a book about the Mandelbrot set" by by Claude Heiland-Allen
- ↑ Automatically finding external angles by Claude Heiland-Allen
- ↑ A Method to Solve the Limitations in Drawing External Rays of the Mandelbrot Set M. Romera,1 G. Pastor, A. B. Orue,1 A. Martin, M.-F. Danca,and F. Montoya
- ↑ Practical interior distance rendering by Claude Heiland-Allen




