Fractals/Mathematics/group/Basilica group

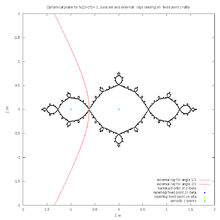
Basilica JUlia set and external rays

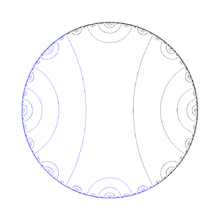
Lamination of Basilica Julia set

NucleusMachine(BasilicaGroup)

Basilica group is :^[1]

• group defined by automatum
• the iterated monodromy group of the polynomial ^[2]
• related with Basilica Julia set : "the scaling limit of the Schreier graphs of its action on level n of T is the basilica"^[3]

Computation

The critical points of the polynomial are and .

The the postcritical set is

FR

predefined by FR package of GAP CAS. Here BinaryKneadingGroup("1") is BasilicaGroup.

gap> BinaryKneadingGroup(1/3)=BasilicaGroup;
true

or :

gap> B := FRGroup("a=<1,b>(1,2)","b=<1,a>",IsFRMealyElement);
<state-closed group over [ 1, 2 ] with 2 generators>
gap> AssignGeneratorVariables(B);
#I  Assigned the global variables [ "a", "b" ]
gap> B=BasilicaGroup;
#I  \=: converting second argument to FR element
#I  \<: converting second argument to FR element
#I  \<: converting second argument to FR element
#I  \=: converting second argument to FR element
#I  \=: converting second argument to FR element
#I  \<: converting second argument to FR element
#I  \<: converting second argument to FR element
#I  \=: converting second argument to FR element
#I  \=: converting first argument to FR element
#I  \=: converting first argument to FR element
#I  \=: converting first argument to FR element
#I  \=: converting first argument to FR element
#I  \=: converting first argument to FR element
#I  \=: converting first argument to FR element
#I  \=: converting first argument to FR element
#I  \=: converting first argument to FR element
true

gap> Size(BasilicaGroup);
infinity
gap> GeneratorsOfGroup(BasilicaGroup);
[ a, b ]
gap> Alphabet(BasilicaGroup);
[ 1, 2 ]
gap> KnownAttributesOfObject(BasilicaGroup);
[ "Name", "Representative", "OneImmutable", "GeneratorsOfMagma", "GeneratorsOfMagmaWithInverses", "MultiplicativeNeutralElement", "UnderlyingFRMachine", "Correspondence", 
"AlphabetOfFRSemigroup", "NucleusOfFRSemigroup", "FRGroupPreImageData", "KneadingSequence", "Alphabet" ]
gap> KnownPropertiesOfObject(BasilicaGroup);
[ "IsDuplicateFree", "IsAssociative", "IsSimpleSemigroup", "IsFinitelyGeneratedGroup", "IsStateClosed", "IsBoundedFRSemigroup", "IsAmenableGroup" ]
gap> KneadingSequence(BasilicaGroup);
[/ '1', '*' ]

References

1. ↑ A Thompson Group for the Basilica by James Belk, Bradley Forrest
2. ↑ R. I. Grigorchuk and A. Zuk (2002a). On a torsion-free weakly branch group defined by a three state automaton. Internat. J. Algebra Comput., 12(1-2):223–246. International Conference on Geometric and Combinatorial Methods in Group Theory and Semigroup Theory (Lincoln, NE, 2000).
3. ↑ Amenability via random walks Laurent Bartholdi and Balint Virag May 19, 2003