Various types of dynamics needs various algorithms

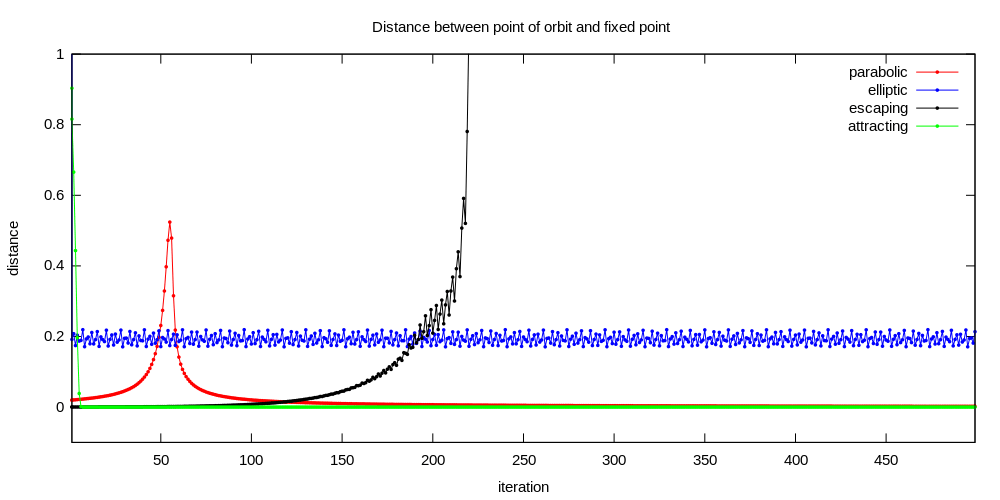
Types

Classification of Julia sets according to :

• topology of filled Julia set's interior
• local dynamics near periodic points
• connectedness
• position of parameter c on the parameter plane ^[1]

Filled Julia set can have :

• an non-empty interior ( Julia set is connected )
  • parabolic: filled Julia set have parabolic cycle ( c is on boundary of hyperbolic componnent )
  • Siegel : filled Julia sets containing Siegel disc. Julia set can be locally connected or not. That depends on the rotation number. ( c is on boundary of hyperbolic component )
  • attracting : filled Julia set have attracting cycle ( c is inside hyperbolic component )
  • superattracting : filled Julia set have superattracting cycle( c is in the center of hyperbolic component ). Examples : Airplane Julia set, Douady's Rabbit, Basillica.
• empty interior
  • disconnected ( c is outside of Mandelbrot set )^[2]
  • connected
    • Cremer Julia sets ( c is on boundary of hyperbolic component , Julia set is connected but not locally connected)
    • dendrits ( Julia set is connected and locally connected ). Examples :
      • Misiurewicz Julia sets (c is a Misiurewicz point )
      • Feigenbaum Julia sets ( )
      • others which have no description

Models of Julia set

Lamination of rabbit Julia set

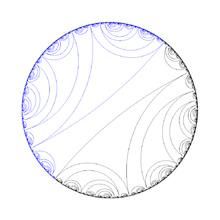
• Thurston’s invariant lamination of Julia set ^[3]
• graph of Julia set ^[4][5]
  • Schreier graphs
• spine of Julia set
• disked tree model ( Hubbard trees serve as a model for Julia sets of post-critically finite polynomials^[6])
• Douady’s pinched disk model
• Milnor’s orbit portraits

Algorithms

"... a single algorithm for computing all quadratic Julia sets does not exist." ^[7]

Types

• Escape time ( attraction time to infinity ( attractor for all polynomials )
• attraction time to finite attractor inside filled Julia set )
• estimation of distance to Julia set ( DEM/J )
• Inverse Iteration Method = IIM/J
• Testing equicontinouty by Michael Becker ^[8]
• orbit traps ^[9][10]

equicontinouty

"The Julia set of f then is the set of all points of G, at which this sequence of iterated functions is not equicontinous. The Fatou set is its complement. Laxly said the action of the iterated functions on near points is examined. Places, where points, which are near enough, remain near during iterations, belong to the Fatou set. Places, where points, as near they may be, are teared apart, belong to the Julia set. In the following I only consider functions, which map the Riemann sphere, i.e. the complex plane with an ideal point "infinity" added, to itself. The Julia sets are white, the Fatou sets black." Michael Becker

points

"We know the periodic points are dense in the Julia set, but in the case of weird ones (like the ones with Cremer points, or even some with Siegel disks where the disk itself is very 'deep' within the Julia set, as measured by the external rays), the periodic points tend to avoid certain parts of the Julia set as long as possible. This is what causes the 'inverse method' of rendering images of Julia sets to be so bad for those cases." ( answered Oct 26 '14 at 14:52 by Jacques Carette )^[11]

References

1. ↑ math.stackexchange question : classification-of-points-in-the-mandelbrot-set
2. ↑ images of disconnected Julia sets
3. ↑ Simple topological models of Julia sets by L. Oversteegen
4. ↑ Combinatorial Julia Sets (1) By Jim Belk
5. ↑ Jacek Skryzalin: On Quadratic Mappings With and Attracting Cycle
6. ↑ Eugene1806's Blog
7. ↑ Computability of Julia sets by Mark Braverman, Michael Yampolsky
8. ↑ Some Julia sets by Michael Becker, 6/2003. Last modification: 2/2004.
9. ↑ orbit traps example video
10. ↑ orbit traps making
11. ↑ Clustering of periodic points for a polynomial iteration of C