Engineering Tables/Trigonometric Identities

< Engineering Tables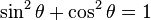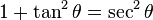

$\sin^2 \theta + \cos^2 \theta = 1$	$1+ \tan^2 \theta = \sec^2 \theta$
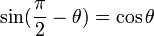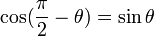$\sin ( \frac{ \pi}{2}- \theta)= \cos \theta$	$\cos ( \frac{ \pi}{2}- \theta)= \sin \theta$
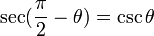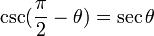$\sec ( \frac{ \pi}{2}- \theta)= \csc \theta$	$\csc ( \frac{ \pi}{2}- \theta)= \sec \theta$
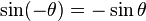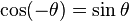$\sin (- \theta)=- \sin \theta$	$\cos (- \theta)= \sin \theta$
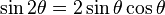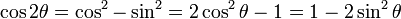$\sin 2 \theta = 2 \sin \theta \cos \theta$	$\cos 2 \theta = \cos^2- \sin^2=2 \cos^2 \theta -1=1-2 \sin^2 \theta$
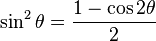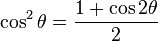$\sin^2 \theta= \frac{1- \cos 2 \theta}{2}$	$\cos^2 \theta= \frac{1+ \cos 2 \theta}{2}$
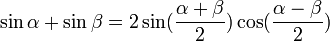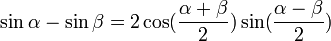$\sin \alpha + \sin \beta = 2 \sin (\frac{ \alpha + \beta}{2}) \cos (\frac{ \alpha - \beta}{2})$	$\sin \alpha - \sin \beta = 2 \cos (\frac{ \alpha + \beta}{2}) \sin (\frac{ \alpha - \beta}{2})$
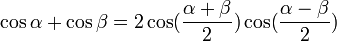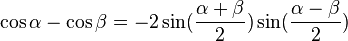$\cos \alpha + \cos \beta = 2 \cos (\frac{ \alpha + \beta}{2}) \cos (\frac{ \alpha - \beta}{2})$	$\cos \alpha - \cos \beta = -2 \sin (\frac{ \alpha + \beta}{2}) \sin (\frac{ \alpha - \beta}{2})$
$\sin \alpha \sin \beta = \frac{1}{2}[\cos( \alpha - \beta) - \cos( \alpha + \beta)]$	$\cos \alpha \cos \beta = \frac{1}{2}[\cos( \alpha - \beta) + \cos( \alpha + \beta)]$
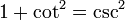$\sin \alpha \cos \beta = \frac{1}{2}[\sin( \alpha + \beta) + \sin( \alpha - \beta)]$	$1+ \cot^2= \csc^2$
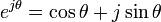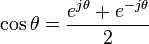$e^{j \theta}= \cos \theta + j \sin \theta$	$\cos \theta = \frac{e^{j \theta} + e^{-j \theta} } {2}$
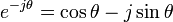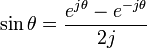$e^{-j \theta} = \cos \theta - j\sin \theta$	$\sin \theta = \frac{e^{j \theta} - e^{-j \theta}} {2j}$
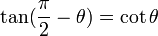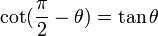$\tan ( \frac{ \pi}{2} - \theta)= \cot \theta$	$\cot ( \frac{ \pi}{2}- \theta)= \tan \theta$
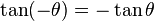$\tan (- \theta)= -\tan \theta$
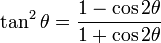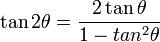$\tan^2 \theta= \frac{1- \cos 2 \theta}{1+ \cos 2 \theta}$	$\tan 2 \theta= \frac{2 \tan \theta}{1-tan^2 \theta}$

This article is issued from Wikibooks. The text is licensed under Creative Commons - Attribution - Sharealike. Additional terms may apply for the media files.