Engineering Tables/Laplace Transform Table 2
< Engineering Tables| ID | Function | Time domain 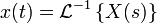 |
Laplace domain 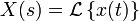 |
Region of convergence for causal systems | ||
|---|---|---|---|---|---|---|
| 1 | Ideal delay | 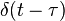 | 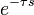 | |||
| 1a | Unit impulse | 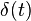 |
 |
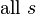 | ||
| 2 | Delayed nth power with frequency shift | 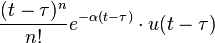 |
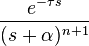 |
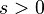 | ||
| 2a | nth Power | 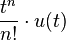 |
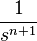 |
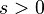 | ||
| 2a.1 | qth Power | 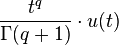 |
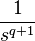 |
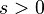 | ||
| 2a.2 | Unit step | 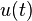 |
 |
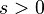 | ||
| 2b | Delayed unit step | 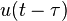 |
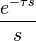 |
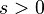 | ||
| 2c | Ramp | 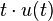 |
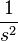 |
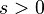 | ||
| 2d | nth Power with frequency shift | 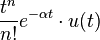 |
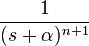 |
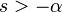 | ||
| 2d.1 | Exponential decay | 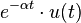 |
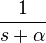 |
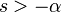 | ||
| 3 | Exponential approach |  |
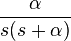 |
 | ||
| 4 | Sine | 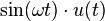 |
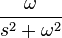 |
 | ||
| 5 | Cosine | 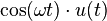 |
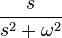 |
 | ||
| 6 | Hyperbolic sine | 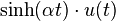 |
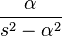 |
 | ||
| 7 | Hyperbolic cosine | 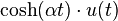 |
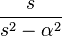 |
 | ||
| 8 | Exponentially-decaying sine | 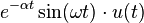 |
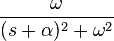 |
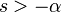 | ||
| 9 | Exponentially-decaying cosine | 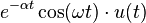 |
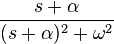 |
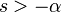 | ||
| 10 | nth Root | ![\sqrt[n]{t} \cdot u(t)](../I/m/486b3056c275d0abfe2730f87a747f9f.png) |
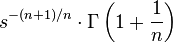 |
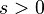 | ||
| 11 | Natural logarithm | 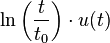 |
![- { t_0 \over s} \ [ \ \ln(t_0 s)+\gamma \ ]](../I/m/6e8a76493bbae4b1d845742deeebdb01.png) |
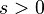 | ||
| 12 | Bessel function of the first kind, of order n |
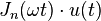 |
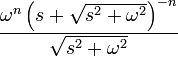 |
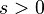 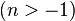 | ||
| 13 | Modified Bessel function of the first kind, of order n |
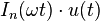 |
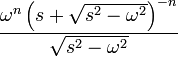 |
 | ||
| 14 | Bessel function of the second kind, of order 0 |
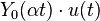 |
||||
| 15 | Modified Bessel function of the second kind, of order 0 |
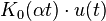 |
||||
| 16 | Error function | 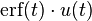 |
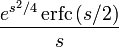 |
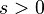 | ||
Explanatory notes:
| ||||||
This article is issued from Wikibooks. The text is licensed under Creative Commons - Attribution - Sharealike. Additional terms may apply for the media files.
 represents the Heaviside step function.
represents the Heaviside step function.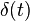 represents the Dirac delta function.
represents the Dirac delta function.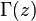 represents the Gamma function.
represents the Gamma function.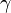 is the Euler-Mascheroni constant.
is the Euler-Mascheroni constant. , a real number, typically represents time,
, a real number, typically represents time,  is the complex angular frequency.
is the complex angular frequency.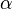 ,
, 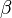 ,
, 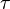 , and
, and 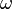 are real numbers.
are real numbers. 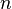 is an integer.
is an integer.