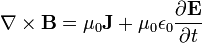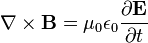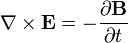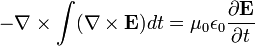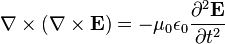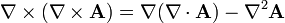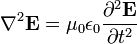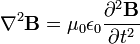Electrodynamics/Electromagnetic Radiation

How Maxwell Fixed Ampère's Law

When Maxwell was looking at Ampere's Law, he realised that changing electric fields acted like currents, but this was not present in its current form. So Maxwell added it, and the full version of Ampere's Law became:

\nabla \times \mathbf {B} = \mu_0 \mathbf {J} + \mu_0 \epsilon_0 \frac{\partial \mathbf{E}}{\partial t}

This realization came about due to the fact that previously Ampere's law was not compatible with the conservation of charge/continuity equation. Upon taking the divergence of Ampere's original equation, it is found that the divergence of the current is equal to zero, which is not in accordance with the conservation of charge. This paradox was resolved through the introduction of the displacement current.

Using The New Law

When we look at this in a vacuum and combine it to Faraday's Law of Induction:

\nabla \times \mathbf {B} = \mu_0 \epsilon_0 \frac{\partial \mathbf{E}}{\partial t}

\nabla \times \mathbf{E} = -\frac{\partial \mathbf{B}} {\partial t}

And then we solve this, we can see that it's a wave travelling with velocity c.

Sketch Proof

\nabla \times \mathbf{E} = -\frac{\partial \mathbf{B}} {\partial t}

-\int (\nabla \times \mathbf{E}) dt = \mathbf{B}

Substituting this into Ampere's Law gives:

-\nabla \times \int (\nabla \times \mathbf{E}) dt = \mu_0 \epsilon_0 \frac{\partial \mathbf{E}}{\partial t}

\nabla \times (\nabla \times \mathbf{E}) = -\mu_0 \epsilon_0 \frac{\partial^2 \mathbf{E}}{\partial t^2}

We now use the following relation:

\nabla \times (\nabla \times \mathbf{A}) = \nabla(\nabla \cdot \mathbf{A}) - \nabla^2 \mathbf{A}

This gives us:

\nabla^2 \mathbf{E} = \mu_0 \epsilon_0 \frac{\partial^2 \mathbf{E}}{\partial t^2}

And similarly:

\nabla^2 \mathbf{B} = \mu_0 \epsilon_0 \frac{\partial^2 \mathbf{B}}{\partial t^2}

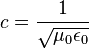This should look familiar to you, it's a second derivative with respect to location, equal to a negative second derivative with respect to time. It's a sine function (really imaginary exponential, but it's satisfactory to use sine here). That moves at velocity $c = \frac{1}{\sqrt{\mu_0 \epsilon_0}}$ .

And experimental values of these numbers gave results extremely close to the observed value of speed of light.

This velocity is so nearly that of light, that it seems we have strong reason to conclude that light itself (including radiant heat, and other radiations if any) is an electromagnetic disturbance in the form of waves propagated through the electromagnetic field according to electromagnetic laws. --Maxwell 1865

How do we really know light is an electromagnetic wave?

Just the fact that the speed comes out correct and the fact that light has wave behaviors (interference and diffraction) is not enough -- after all, gravitational waves would also have these properties. Perhaps the most compelling evidence that light is an electromagnetic wave is that

The reflection of light from conductors and from dielectrics behaves as one expects from Maxwell's equations
The polarization of light produced by reflection and scattering is as one expects from Maxwell
High intensity lasers can cause air and other (dielectric) materials to "break down". This looks like a tiny spark, just as one would expect if light is made of electric and magnetic fields, with the electric field causing dielectric breakdown.
There is a continuum of radiation types, from radio waves, which we know are electromagnetic because we fabricate it (and capture their charges with antennas), to light, through microwaves, far infrared, near infrared, light (and beyond). These radiation types exhibit properties continuously and smoothly varying with their wavelength, without jumps.

This article is issued from Wikibooks. The text is licensed under Creative Commons - Attribution - Sharealike. Additional terms may apply for the media files.