Differential Geometry/Osculating Plane

< Differential Geometry

The term osculating plane, which was first used by Tinseau in 1780, of a curve C parametrized by a function f(t) at a point f(a) is the plane that is approached when it is spanned by two vectors f(x)-f(a) and f(y)-f(a) when x and y both approach a.

First, assume that the curve C is at least of class 2.

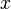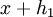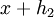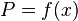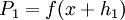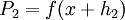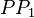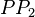Then consider the points $x$ , $x+h_1$ , and $x+h_2$ and consider the points $P=f(x)$ , $P_1=f(x+h_1)$ , and $P_2=f(x+h_2)$ . The segments $PP_1$ and $PP_2$ are the vectors 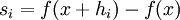$s_i=f(x+h_i)-f(x)$ . If these vectors are linearly independent, then they span a plane.

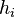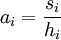We can divide each of those vectors by $h_i$ , meaning that the plane is also spanned by the vectors $a_i=\frac{s_i}{h_i}$ .

We can also replace the second vector by 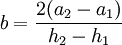$b=\frac{2(a_2-a_1)}{h_2-h_1}$ and it is easy to see that 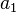$a_1$ and w span the same plane as the original vectors.

Using Taylor's formula, we get

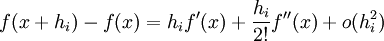$f(x+h_i)-f(x)=h_if'(x)+\frac{h_i}{2!}f''(x)+o(h_i^2)$ .

This indicates that 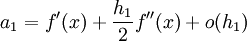$a_1=f'(x)+\frac{h_1}{2}f''(x)+o(h_1)$ and that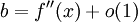 $b=f''(x)+o(1)$

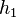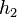Thus, as both $h_1$ and $h_2$ approach 0, 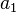$a_1$ approaches f'(x) and 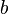$b$ approaches f(x). The osculating plane is consequently spanned by f'(x) and f(x), and consequently contains the tangent line.

Consider the position vector 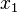$x_1$ of any point on the osculating plane.

Then it is obvious that the following scalar triple product is equal to 0:

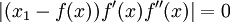$|(x_1-f(x))f'(x)f''(x)|=0$ .

The intersection of the osculating plane and the normal plane is called the principal normal line.

If it happens to be the case that f'(x) and f(x) are linearly dependent, then we can consider every plane containing the tangent line to be the osculating plane.

This article is issued from Wikibooks. The text is licensed under Creative Commons - Attribution - Sharealike. Additional terms may apply for the media files.