Differential Geometry/Frenet-Serret Formulae

< Differential Geometry

The derivatives of the vectors t, p, and b can be expressed as a linear combination of these vectors. The formulae for these expressions are called the Frenet-Serret Formulae. This is natural because t, p, and b form an orthogonal basis for a three-dimensional vector space.

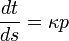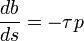Of course, we know already that $\frac{dt}{ds}=\kappa p$ and $\frac{db}{ds}=-\tau p$ so it remains to find 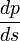$\frac{dp}{ds}$ . First, we differentiate 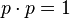$p \sdot p = 1$ to obtain 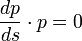$\frac{dp}{ds}\sdot p = 0$ so it takes on the form 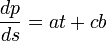$\frac{dp}{ds}=at+cb$ . We take the dot product of this with t to obtain 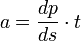$a=\frac{dp}{ds}\sdot t$ . 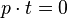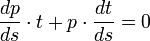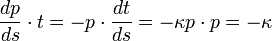Taking the derivative of $p\sdot t=0$ , we get $\frac{dp}{ds}\sdot t + p \sdot \frac{dt}{ds} = 0$ or $\frac{dp}{ds}\sdot t=-p \sdot \frac{dt}{ds} = -\kappa p \sdot p = -\kappa$ . Also, taking the dot product of 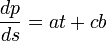$\frac{dp}{ds}=at+cb$ with b, we obtain 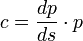$c=\frac{dp}{ds}\sdot p$ . Taking the derivative of 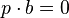$p\sdot b=0$ , we get 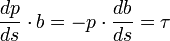$\frac{dp}{ds}\sdot b=-p\sdot\frac{db}{ds}=\tau$ . 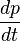Thus, we arrive at the following expression for $\frac{dp}{dt}$ :

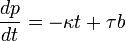$\frac{dp}{dt}=-\kappa t+\tau b$ .

This formula, combined with the previous two formulae, are together called the Frenet-Serret Formulae and they can be represented by a skew-symmetric matrix.

This article is issued from Wikibooks. The text is licensed under Creative Commons - Attribution - Sharealike. Additional terms may apply for the media files.