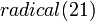Conic Sections/Circle

Definition

The circle is the simplest and best known conic section. As a conic section, the circle is the intersection of a plane perpendicular to the cone's axis.

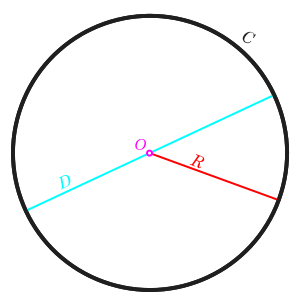
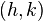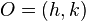The geometric definition of a circle is the locus of all points a constant distance $r$ from a point $(h,k)$ and forming the circumference (C). The distance $r$ is the radius (R) of the circle, and the point $O = (h,k)$ is the circle's center also spelled as centre. The diameter (D) is twice the length of the radius.

Circle illustration with circumference (C) in black, diameter (D) in cyan, radius (R) in red, and centre or origin (O) in magenta.

Equations

General Form

The general equation for a circle with center 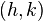$(h,k)$ and radius $r$ is

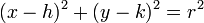(x-h)^2+(y-k)^2=r^2

In the simplest case of a circle whose center is at the origin, the equation is simply a restatement of the Pythagorean Theorem:

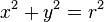
x^2 + y^2 = r^2

General form

The general form of a circle equation is

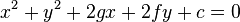x^2+y^2+2gx+2fy+c=0

, where

<-g,-f> is the center of the circle.

Polar Coordinates

In the case of a circle centered at the origin, the polar equation of a circle is very simple because polar coordinates are essentially based on circles. For a circle with radius 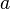$a$ ,

r = a

In the more complicated case of a circle with an arbitrary location, the equation is

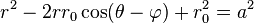r^2 - 2 r r_0 \cos(\theta - \varphi) + r_0^2 = a^2

,
where

r_0

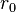is the distance from the circle's center to the origin and

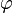\varphi

is the angle pointing to the circle.

There are many cases that allow the equation to be simplified. If a point on the circle is touching the origin, its polar equation may consist of a single trig function.

.....

Parametric Equations

When the circle's equation is parametrized with respect to $t$ , the equation becomes

x=h + r \cos t

y=k + r \sin t

Example

Find the center and the radius of the following circle: x²+y²+8x-10y+20=0 find by:

x²+y²+8x-10y+20=0
x²+y²+8x-10y= - 20
(x²+8x)+(y²-10y)= - 20
+16 +25 +16+25
(x²+8x+16)+(y²-10y+25)=21
(x+4)²+(y-5)²=21

Thus:
C(-4,5) radius= $radical(21)$

This article is issued from Wikibooks. The text is licensed under Creative Commons - Attribution - Sharealike. Additional terms may apply for the media files.