Arithmetic/Multiplying Fractions

Multiplying fractions

To multiply two fractions:

multiply the numerators to get the new numerator, and
multiply the denominators to get the new denominator.

For instance,

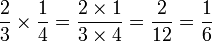\frac{2}{3} \times \frac{1}{4} = \frac{2 \times 1}{3 \times 4} = \frac{2}{12} = \frac{1}{6}

Dividing fractions

To divide one fraction by another one, flip numerator and denominator of the second one, and then multiply the two fractions. The flipped-over fraction is called the multiplicative inverse or reciprocal.

For instance,

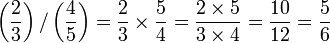\left(\frac{2}{3}\right) / \left(\frac{4}{5}\right) = \frac{2}{3} \times \frac{5}{4} = \frac{2 \times 5}{3 \times 4} = \frac{10}{12} = \frac{5}{6}

To simplify a compound fraction, like 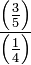$\frac{\left(\frac{3}{5}\right)}{\left(\frac{1}{4}\right)}$ , just remember that a fraction is the same as division, and divide (3/5) ÷ (1/4), which comes to 12/5.

This article is issued from Wikibooks. The text is licensed under Creative Commons - Attribution - Sharealike. Additional terms may apply for the media files.