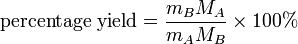A-level Chemistry/OCR (Salters)/Yield

< A-level Chemistry < OCR (Salters)

Percentage yield is a useful way of saying how much of a reactant has been successfully converted to product in a chemical reaction.

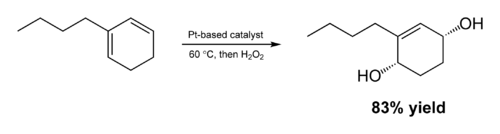
from J. Am. Chem. Soc. (2009) 131 (26), 9134–9135

If all the reactants become products, the percentage yield is 100%. If half the reactants become products and the rest become by-products or don't react at all, the percentage yield is 50%. If none of the reactants end up as products, the percentage yield is 0%.

In a nutshell

For a reaction where, ideally, one mole of starting material A is converted to one mole of products B, i.e. A→ B:

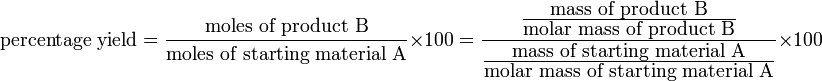
$\mbox{percentage yield} = \frac{ \mbox{moles of product B} }{ \mbox{moles of starting material A} } \times 100 = \frac{\frac{\mbox{mass of product B}}{\mbox{molar mass of product B}}}{\frac{\mbox{mass of starting material A}}{\mbox{molar mass of starting material A}}} \times 100$

Importance

Percentage yield is important because:

chemical reactions very often form by-products as well as the intended product
in most reactions, not all of the reactants actually react

What is left at the end of a reaction is thus typically a mixture of product and impurities (impurities being by-products and unreacted starting materials).

Achieving high percentage yields is particularly important in industries that rely on organic synthesis, such as the pharmaceutical industry. It may require tens of steps to synthesise a drug molecule — if there are ten steps, each of which has a percentage yield of 90%, the overall yield is only 35%:

90% × 90% × 90% × 90% × 90% × 90% × 90% × 90% × 90% × 90% = (90%)¹⁰ = 35%

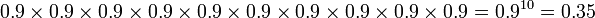
$0.9 \times 0.9 \times 0.9 \times 0.9 \times 0.9 \times 0.9 \times 0.9 \times 0.9 \times 0.9 \times 0.9 = 0.9^{10} = 0.35$

In certain reactions (such as the Haber process) reactants can be recycled, in which case high yields are less of an issue.

Percentage yield formula

There is a simple formula you can use to calculate the percentage yield of a reaction A → B:

$\mbox{percentage yield} = \frac{ \mbox{moles of product B} }{ \mbox{moles of reactant A} } \times 100$

Percentage yield effectively states the percentage of moles of reactant that become product — although this definition only applies if one mole of reactant ideally forms one mole of product, i.e. 1:1 stoichiometry.

In practice, the number of moles of A and B involved have to be calculated using the masses, m and molar masses, M, of A and B.

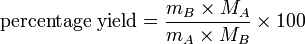
$\mbox{percentage yield} = \frac{ m_B \times M_A }{ m_A \times M_B } \times 100$

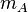$m_A \,\!$ stands for the mass of substance A in g
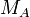$M_A \,\!$ stands for the molar mass of substance A in g mol⁻¹
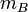$m_B \,\!$ stands for the mass of substance B in g
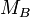$M_B \,\!$ stands for the molar mass of substance B in g mol⁻¹

In words, this is:

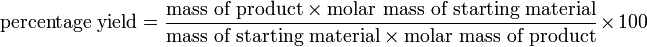
$\mbox{percentage yield} = \frac{ \mbox{mass of product} \times \mbox{molar mass of starting material} }{ \mbox{mass of starting material} \times \mbox{molar mass of product} } \times 100$

This can also be written as:

$\mbox{percentage yield} = \frac{ \mbox{mass of product} \div \mbox{molar mass of product} }{ \mbox{mass of starting material} \div \mbox{molar mass of starting material} } \times 100$

Another way of writing the same this is given below, but this form is a little untidy:

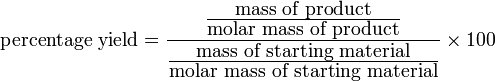
$\mbox{percentage yield} = \frac{\frac{\mbox{mass of product}}{\mbox{molar mass of product}}}{\frac{\mbox{mass of starting material}}{\mbox{molar mass of starting material}}} \times 100$

Derivation of formula

Notation

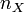$n_X\,\!$ means number of moles of substance X

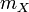$m_X\,\!$ means mass of substance X

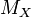$M_X\,\!$ means molar mass of substance X

Definition of yield

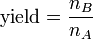
$\mbox{yield} = \frac{ n_B }{ n_A }$

Definition of percentage yield

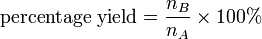
$\mbox{percentage yield} = \frac{ n_B }{ n_A } \times 100%$

Expressing moles in terms of mass and RFM

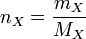$n_X = \frac{m_X}{M_X}$ , which you may know as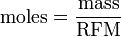 $\mbox{moles} = \frac{\mbox{mass}}{\mbox{RFM}}$

therefore, wherever 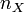$n_X \,\!$ appears, it can be replaced with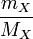 $\frac{m_X}{M_X}$

thus 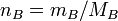$n_B = m_B / M_B \,\!$

and 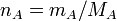$n_A = m_A / M_A \,\!$

Rewriting the yield formula in terms of mass and RFM

substituting the above equations into the formula for percentage yield

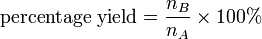
$\mbox{percentage yield} = \frac{ n_B }{ n_A } \times 100%$

gives

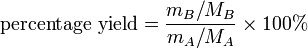
$\mbox{percentage yield} = \frac{ m_B / M_B }{ m_A / M_A } \times 100%$

which can be rearranged to give

$\mbox{percentage yield} = \frac{ m_B M_A }{ m_A M_B } \times 100%$

This article is issued from Wikibooks. The text is licensed under Creative Commons - Attribution - Sharealike. Additional terms may apply for the media files.